Advertisements
Advertisements
Question
Show that in a right angled triangle, the hypotenuse is the longest side.
Solution

Let us consider a right-angled triangle ABC, right-angled at B.
In ΔABC,
∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)
∠A + 90º + ∠C = 180°
∠A + ∠C = 90°
Hence, the other two angles have to be acute (i.e., less than 90º).
∴ ∠B is the largest angle in ΔABC.
⇒ ∠B > ∠A and ∠B > ∠C
⇒ AC > BC and AC > AB
[In any triangle, the side opposite to the larger (greater) angle is longer.]
Therefore, AC is the largest side in ΔABC.
However, AC is the hypotenuse of ΔABC. Therefore, hypotenuse is the longest side in a right-angled triangle.
APPEARS IN
RELATED QUESTIONS
In the given figure, ∠B < ∠A and ∠C < ∠D. Show that AD < BC.

Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Name the smallest angle in each of these triangles:
In ΔPQR, PQ = 8.3cm, QR = 5.4cm and PR = 7.2cm
Name the smallest angle in each of these triangles:
In ΔXYZ, XY = 6.2cm, XY = 6.8cm and YZ = 5cm
ABCD is a quadrilateral in which the diagonals AC and BD intersect at O. Prove that AB + BC + CD + AD < 2(AC + BC).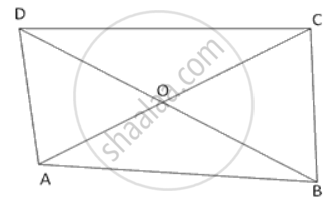
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
ABCD is a trapezium. Prove that: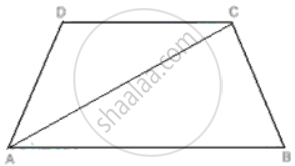
CD + DA + AB > BC.
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PQ > PS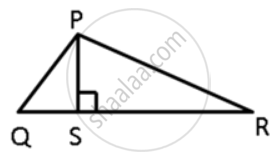
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
