Advertisements
Advertisements
Question
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
Solution
In ΔABC, we have
AB + BC > AC ...(i)
In ΔACD, we have
AD + CD > AC ...(ii)
Adding (i) and (ii), we get
AB + BC + AD + CD > 2AC.
APPEARS IN
RELATED QUESTIONS
Show that in a right angled triangle, the hypotenuse is the longest side.
ABC is a triangle. Locate a point in the interior of ΔABC which is equidistant from all the vertices of ΔABC.
In the following figure, write BC, AC, and CD in ascending order of their lengths.
Name the smallest angle in each of these triangles:
In ΔPQR, PQ = 8.3cm, QR = 5.4cm and PR = 7.2cm
ABCD is a trapezium. Prove that: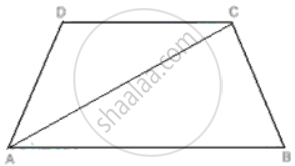
CD + DA + AB > BC.
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that: SN < SR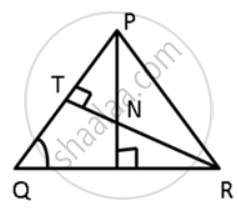
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PQ > PS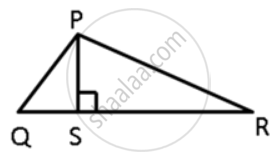
In ΔPQR, PS ⊥ QR ; prove that: PQ + PR > QR and PQ + QR >2PS.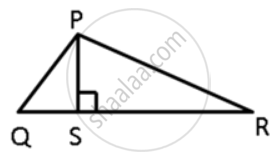
In ΔPQR is a triangle and S is any point in its interior. Prove that SQ + SR < PQ + PR.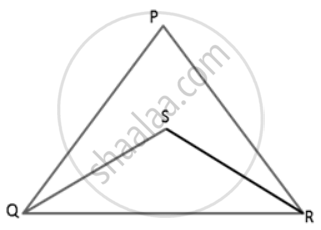
In ΔABC, D is a point in the interior of the triangle. Prove that DB + DC < AB + AC.
