Advertisements
Advertisements
Question
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PQ > PS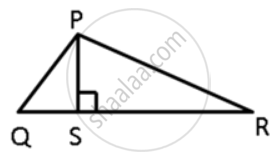
Solution
In ΔPQS,
PS < PQ ....(Of all the straight lines that can be drawn to a given straight line from a point outside it, the perpendicular is the shortest.)
I.e. PQ > PS
Also, QS < QP ....(Of all the straight lines that can be drawn to a given straight line from a point outside it, the perpendicular is the shortest.)
i.e. PQ > QS.
APPEARS IN
RELATED QUESTIONS
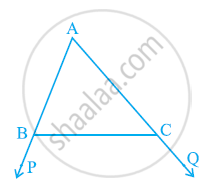
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
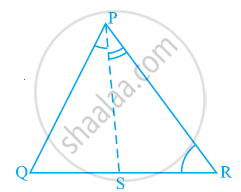
In the given figure, PR > PQ and PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.
Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.
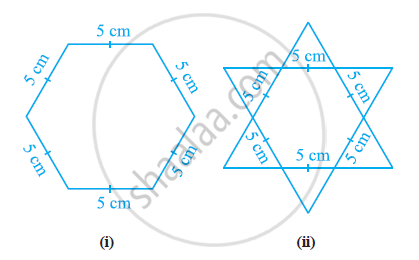
Complete the hexagonal and star shaped rangolies (see the given figures) by filling them with as many equilateral triangles of side 1 cm as you can. Count the number of triangles in each case. Which has more triangles?
Arrange the sides of the following triangles in an ascending order:
ΔDEF, ∠D = 38°, ∠E = 58°.
Name the smallest angle in each of these triangles:
In ΔPQR, PQ = 8.3cm, QR = 5.4cm and PR = 7.2cm
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.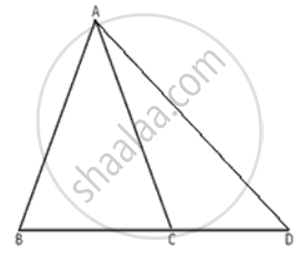
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.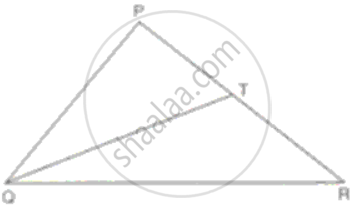
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT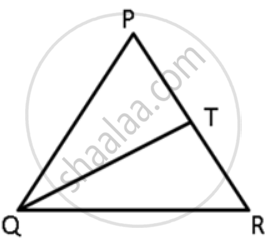
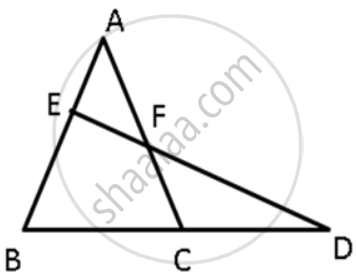
ΔABC in a isosceles triangle with AB = AC. D is a point on BC produced. ED intersects AB at E and AC at F. Prove that AF > AE.