Advertisements
Advertisements
Question
ABCD is a trapezium. Prove that: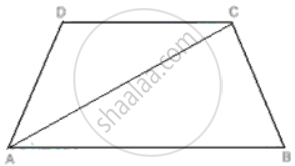
CD + DA + AB > BC.
Solution
In ΔACD, we have
CD + DA > CA
⇒ CD + DA + AB > CA +AB
⇒ CD + DA + AB > BC. ...[∴ AB + AC > BC]
APPEARS IN
RELATED QUESTIONS
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
Name the greatest and the smallest sides in the following triangles:
ΔABC, ∠ = 56°, ∠B = 64° and ∠C = 60°.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
Name the smallest angle in each of these triangles:
In ΔABC, AB = 6.2cm, BC = 5.6cm and AC = 4.2cm
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.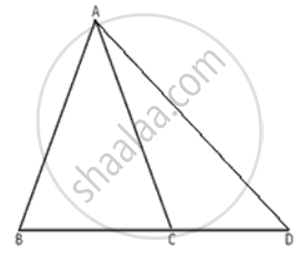
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
In ΔPQR, PR > PQ and T is a point on PR such that PT = PQ. Prove that QR > TR.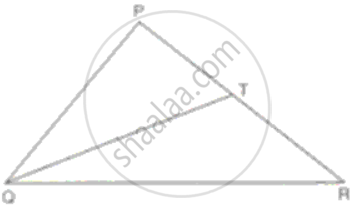
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN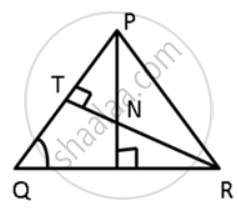
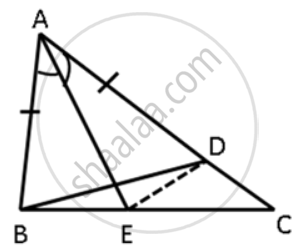
In ΔABC, AE is the bisector of ∠BAC. D is a point on AC such that AB = AD. Prove that BE = DE and ∠ABD > ∠C.