Advertisements
Advertisements
प्रश्न
In the following figure, write BC, AC, and CD in ascending order of their lengths.
उत्तर

In ΔABC,
∠BAC < ∠ABC
BC < AC ....( 1 )
Now, ∠ACB = 180° - ∠ABC - ∠BAC
∠ACB = 180° - 73° - 47°
∠ACB = 60°
Now, ∠ACD = 180° - ∠ACB
∠ACD = 180° - 60° = 120°
Now, in ΔACD,
∠ADC = 180° - ∠ACD - ∠CAD
∠ADC = 180° - 120° - 31°
∠ADC = 29°
Since ∠ADC < ∠CAD, we have
AC < CD ....( 2 )
From ( 1 ) and ( 2 ), we have
BC < AC < CD.
APPEARS IN
संबंधित प्रश्न
Show that in a right angled triangle, the hypotenuse is the longest side.
Show that of all line segments drawn from a given point not on it, the perpendicular line segment is the shortest.
From the following figure, prove that: AB > CD.
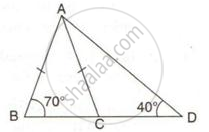
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
In the following figure ; AC = CD; ∠BAD = 110o and ∠ACB = 74o.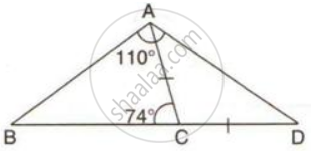
Prove that: BC > CD.
Prove that the hypotenuse is the longest side in a right-angled triangle.
In ABC, P, Q and R are points on AB, BC and AC respectively. Prove that AB + BC + AC > PQ + QR + PR.
ABCD is a trapezium. Prove that:
CD + DA + AB + BC > 2AC.
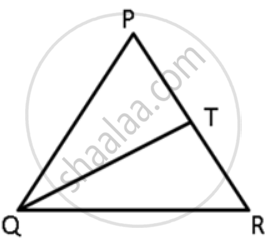
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that: SN < SR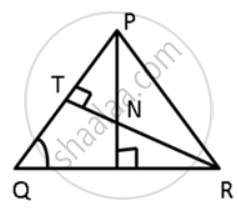
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT