Advertisements
Advertisements
प्रश्न
In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to ______.
विकल्प
80°
40°
50°
100°
उत्तर
In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to 50°.
Explanation:
Given, ∆ABC such that BC = AB and ∠B = 80°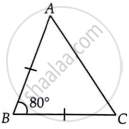
In ∆ABC, AB = BC
⇒ ∠C = ∠A ...(i) [Angles opposite to equal sides are equal]
We know that, the sum of all the angles of a triangle is 180°.
∴ ∠A + ∠B + ∠C = 180°
⇒ ∠A + 80° + ∠A = 180° ...[From equation (i)]
⇒ 2∠A = 180° – 80° = 100°
⇒ ∠A = `(100^circ)/2`
⇒ ∠A = 50°
APPEARS IN
संबंधित प्रश्न
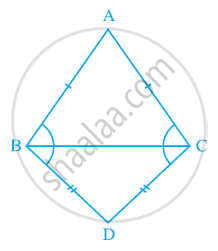
ABC and DBC are two isosceles triangles on the same base BC (see the given figure). Show that ∠ABD = ∠ACD.
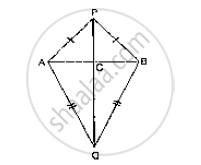
AB is a line seg P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (See Fig. 10.26). Show that the line PQ is perpendicular bisector of AB.
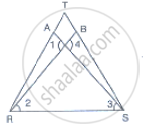
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT
Find the measure of each exterior angle of an equilateral triangle.
Which of the following statements are true (T) and which are false (F) :
If the altitude from one vertex of a triangle bisects the opposite side, then the triangle may be isosceles.
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
Fill the blank in the following so that the following statement is true.
In an isosceles triangle ABC with AB = AC, if BD and CE are its altitudes, then BD is …… CE.
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
In a triangle ABC, if AB = AC and AB is produced to D such that BD = BC, find ∠ACD: ∠ADC.
Is it possible to construct a triangle with lengths of its sides as 9 cm, 7 cm and 17 cm? Give reason for your answer.
