Advertisements
Advertisements
प्रश्न
AB is a line seg P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (See Fig. 10.26). Show that the line PQ is perpendicular bisector of AB.
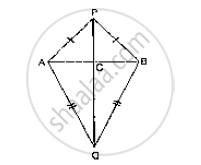
उत्तर
Consider the figure,
We have
AB is a line segment and P,Q are points on opposite sides of AB such that
AP = BP .......(1)
AQ = BQ ........(2)
We have to prove that PQ is perpendicular bisector of AB. Now consider DPAQ and DPBQ,
We have AP = BP [∵ From (1)]
AQ = BQ [∵ From (2)]
And PQ = PQ [Common site]
⇒∠PAQ ≅ ∠PBQ ..….(3) [From SSS congruence]
Now, we can observe that Δ𝐴𝑃𝐵 𝑎𝑛𝑑 Δ𝐴𝐵𝑄 are isosceles triangles.(From 1 and 2)
⟹∠𝑃𝐴𝐵 = ∠𝑃𝐵𝐴 𝑎𝑛𝑑 ∠𝑄𝐴𝐵 = ∠𝑄𝐵𝐴
Now consider ΔPAC and , ΔPBC
C is the point of intersection of AB and PQ.
PA = PB [from (1)]
∠APC = ∠BPC [from (2)]
PC = PC [Common side]
So, from SAS congruency of triangle ΔPAC ≅ ΔPBC
⇒AC = CB and∠PCA = ∠PCB …….(4)
[∵ Corresponding parts of congruent triangles are equal] And also, ACB is line segment
⇒∠ACP + ∠BCP = 180°
But ∠ACP =∠PCB
⇒∠ACP = ∠PCB = 90° ………(5)
We have AC = CB ⇒ C is the midpoint of AB
From (4) and (5)
We can conclude that PC is the perpendicular bisector of AB
Since C is a point on the line PQ, we can say that PQ is the perpendicular bisector of AB.

APPEARS IN
संबंधित प्रश्न
Two lines AB and CD intersect at O such that BC is equal and parallel to AD. Prove that the lines AB and CD bisect at O.
Which of the following statements are true (T) and which are false (F):
Sides opposite to equal angles of a triangle may be unequal
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
Fill the blank in the following so that the following statement is true.
Angle opposite to equal sides of a triangle are .....
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
If the angles of a triangle are in the ratio 2 : 1 : 3, then find the measure of smallest angle.
In a ΔABC, if ∠A = 60°, ∠B = 80° and the bisectors of ∠B and ∠C meet at O, then ∠BOC =
If the bisectors of the acute angles of a right triangle meet at O, then the angle at Obetween the two bisectors is
In a ΔABC, ∠A = 50° and BC is produced to a point D. If the bisectors of ∠ABC and ∠ACDmeet at E, then ∠E =
In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to ______.
