Advertisements
Advertisements
प्रश्न
In \[∆\] ABC , the coordinates of vertex A are (0, - 1) and D (1,0) and E(0,10) respectively the mid-points of the sides AB and AC . If F is the mid-points of the side BC , find the area of \[∆\] DEF.
उत्तर
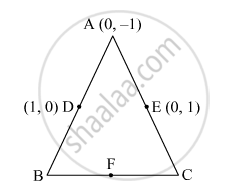
Let the coordinates of B and C be \[\left( x_2 , y_2 \right)\] and \[\left( x_3 , y_3 \right)\] , respectively.
D is the midpoint of AB.
So,
\[\left( 1, 0 \right) = \left( \frac{x_2 + 0}{2}, \frac{y_2 - 1}{2} \right)\]
\[ \Rightarrow 1 = \frac{x_2}{2} \text { and } 0 = \frac{y_2 - 1}{2}\]
\[ \Rightarrow x_2 = 2 \text{ and } y_2 = 1\]
Thus, the coordinates of B are (2, 1).
Similarly, E is the midpoint of AC.
So,
\[\left( 0, 1 \right) = \left( \frac{x_3 + 0}{2}, \frac{y_3 - 1}{2} \right)\]
\[ \Rightarrow 0 = \frac{x_3}{2} \text{ and } 1 = \frac{y_3 - 1}{2}\]
\[ \Rightarrow x_3 = 0 \text{ and } y_3 = 3\]
Thus, the coordinates of C are (0, 3).
Also, F is the midpoint of BC. So, its coordinates are \[\left( \frac{2 + 0}{2}, \frac{1 + 3}{2} \right) = \left( 1, 2 \right)\]
\[\frac{1}{2}\left[ 0\left( 1 - 3 \right) + 2\left( 3 + 1 \right) + 0\left( - 1 - 1 \right) \right]\]
\[ = \frac{1}{2} \times 8\]
\[ = 4 \text{ square units } \]
And the area of \[∆\] DEF is
\[\frac{1}{2}\left[ 1\left( 1 - 2 \right) + 0\left( 2 - 0 \right) + 1\left( 0 - 1 \right) \right]\]
\[ = \frac{1}{2} \times \left( - 2 \right)\]
\[ = 1 \text{ square unit } \left( \text{ Taking the numerical value, as the area cannot be negative } \right)\]
APPEARS IN
संबंधित प्रश्न
Find the centre of the circle passing through (5, -8), (2, -9) and (2, 1).
Find the points of trisection of the line segment joining the points:
5, −6 and (−7, 5),
Show that the points A (1, 0), B (5, 3), C (2, 7) and D (−2, 4) are the vertices of a parallelogram.
Determine the ratio in which the point (-6, a) divides the join of A (-3, 1) and B (-8, 9). Also, find the value of a.
If the point C ( - 2,3) is equidistant form the points A (3, -1) and Bx (x ,8) , find the value of x. Also, find the distance between BC
Show that the points A(6,1), B(8,2), C(9,4) and D(7,3) are the vertices of a rhombus. Find its area.
Points P, Q, R and S divide the line segment joining the points A(1,2) and B(6,7) in five equal parts. Find the coordinates of the points P,Q and R
The base BC of an equilateral triangle ABC lies on y-axis. The coordinates of point C are (0, -3). The origin is the midpoint of the base. Find the coordinates of the points A and B. Also, find the coordinates of another point D such that ABCD is a rhombus.
The abscissa of any point on y-axis is
If (a,b) is the mid-point of the line segment joining the points A (10, - 6) , B (k,4) and a - 2b = 18 , find the value of k and the distance AB.
If \[D\left( - \frac{1}{5}, \frac{5}{2} \right), E(7, 3) \text{ and } F\left( \frac{7}{2}, \frac{7}{2} \right)\] are the mid-points of sides of \[∆ ABC\] , find the area of \[∆ ABC\] .
If points (t, 2t), (−2, 6) and (3, 1) are collinear, then t =
The length of a line segment joining A (2, −3) and B is 10 units. If the abscissa of B is 10 units, then its ordinates can be
Signs of the abscissa and ordinate of a point in the second quadrant are respectively.
Point (3, 0) lies in the first quadrant.
Find the coordinates of the point which lies on x and y axes both.
In which ratio the y-axis divides the line segment joining the points (5, – 6) and (–1, – 4)?
Co-ordinates of origin are ______.
Ryan, from a very young age, was fascinated by the twinkling of stars and the vastness of space. He always dreamt of becoming an astronaut one day. So, he started to sketch his own rocket designs on the graph sheet. One such design is given below :
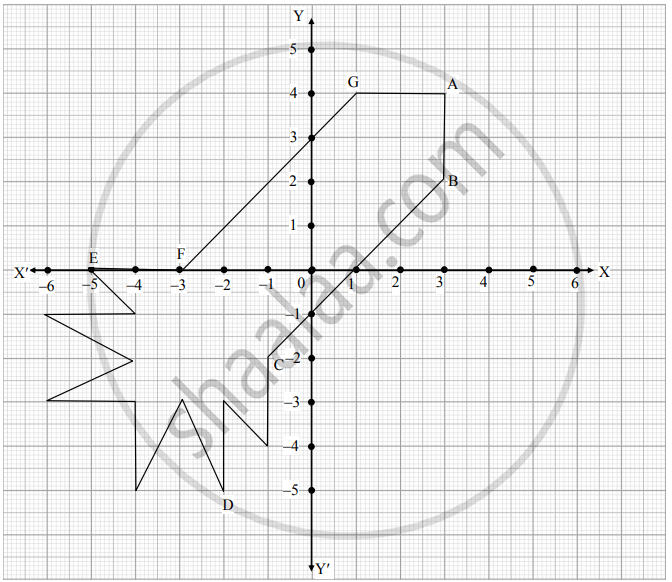
Based on the above, answer the following questions:
i. Find the mid-point of the segment joining F and G. (1)
ii. a. What is the distance between the points A and C? (2)
OR
b. Find the coordinates of the points which divides the line segment joining the points A and B in the ratio 1 : 3 internally. (2)
iii. What are the coordinates of the point D? (1)
