Advertisements
Advertisements
प्रश्न
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADC
उत्तर
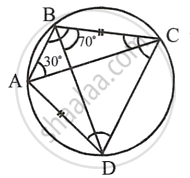
ABCD is a cyclic quadrilateral and AD = BC
∠BAC = 30°, ∠CBD = 70°
∠DAC = ∠CBD ...[Angles in the same segment]
`=>` ∠DAC = 70° ...[∵ CBD = 70°]
`=>` ∠BAD = ∠BAC + ∠DAC = 30° + 70° = 100°
Since the sum of opposite angles of cyclic quadrilateral is supplementary
∠BAD + ∠BCD =180°
`=>` 100°+ ∠BCD = 180° ...[From (1)]
`=>` ∠BCD = 180° – 100° = 80°
Since, AD = BC, ∠ACD = ∠BDC ...[Equal chords subtends equal angles]
But ∠ACB = ∠ADB ...[Angles in the same segment]
∴ ∠ACD + ∠ACB = ∠BDC + ∠ADB
`=>` ∠BCD = ∠ADC = 80°
But in ∆BCD,
∠CBD + ∠BCD + ∠BDC = 180° ...[Angles af a triangle]
`=>` 70° + 80° + ∠BDC = 180°
`=>` 150° + ∠BDC = 180°
∴ ∠BDC = 180° – 150° = 30°
`=>` ∠ACD = 30° ...[∵ ∠ACD = ∠BDC]
∴ ∠BCA = ∠BCD – ∠ACD = 80° – 30° = 50°
Since the sum of opposite angles of cyclic quadrilateral is supplementary
∠ADC + ∠ABC = 180°
`=>` 80° + ∠ABC = 180°
`=>` ∠ABC = 180° – 80° = 100°
APPEARS IN
संबंधित प्रश्न
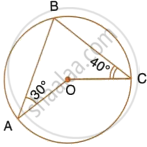
In the given figure, O is the centre of the circle. ∠OAB and ∠OCB are 30° and 40° respectively. Find ∠AOC . Show your steps of working.
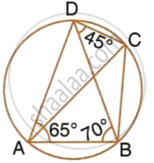
In the given figure, ∠BAD = 65°, ∠ABD = 70°, ∠BDC = 45°
- Prove that AC is a diameter of the circle.
- Find ∠ACB.

In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
The given figure shows a circle with centre O and ∠ABP = 42°.

Calculate the measure of:
- ∠PQB
- ∠QPB + ∠PBQ
In the given figure, AB = AD = DC = PB and ∠DBC = x°. Determine, in terms of x :
- ∠ABD,
- ∠APB.
Hence or otherwise, prove that AP is parallel to DB.
In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.

In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°, find the number of degrees in ∠ABC.

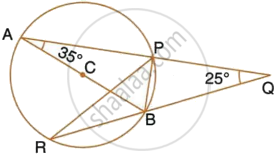
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PBR
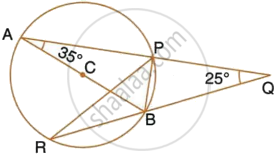
AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that :
`∠QPR = 90^circ - 1/2 ∠BAC`