Advertisements
Advertisements
Question
In cyclic quadrilateral ABCD; AD = BC, ∠BAC = 30° and ∠CBD = 70°; find:
- ∠BCD
- ∠BCA
- ∠ABC
- ∠ADC
Solution
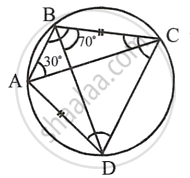
ABCD is a cyclic quadrilateral and AD = BC
∠BAC = 30°, ∠CBD = 70°
∠DAC = ∠CBD ...[Angles in the same segment]
`=>` ∠DAC = 70° ...[∵ CBD = 70°]
`=>` ∠BAD = ∠BAC + ∠DAC = 30° + 70° = 100°
Since the sum of opposite angles of cyclic quadrilateral is supplementary
∠BAD + ∠BCD =180°
`=>` 100°+ ∠BCD = 180° ...[From (1)]
`=>` ∠BCD = 180° – 100° = 80°
Since, AD = BC, ∠ACD = ∠BDC ...[Equal chords subtends equal angles]
But ∠ACB = ∠ADB ...[Angles in the same segment]
∴ ∠ACD + ∠ACB = ∠BDC + ∠ADB
`=>` ∠BCD = ∠ADC = 80°
But in ∆BCD,
∠CBD + ∠BCD + ∠BDC = 180° ...[Angles af a triangle]
`=>` 70° + 80° + ∠BDC = 180°
`=>` 150° + ∠BDC = 180°
∴ ∠BDC = 180° – 150° = 30°
`=>` ∠ACD = 30° ...[∵ ∠ACD = ∠BDC]
∴ ∠BCA = ∠BCD – ∠ACD = 80° – 30° = 50°
Since the sum of opposite angles of cyclic quadrilateral is supplementary
∠ADC + ∠ABC = 180°
`=>` 80° + ∠ABC = 180°
`=>` ∠ABC = 180° – 80° = 100°
APPEARS IN
RELATED QUESTIONS
A triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that:
- ∠ABC = 2∠APQ,
- ∠ACB = 2∠APR,
- `∠QPR = 90^circ - 1/2 ∠BAC`.
The given figure shows a circle with centre O and ∠ABP = 42°.

Calculate the measure of:
- ∠PQB
- ∠QPB + ∠PBQ
In a cyclic-trapezium, the non-parallel sides are equal and the diagonals are also equal. Prove it.
If I is the incentre of triangle ABC and AI when produced meets the circumcircle of triangle ABC in point D. If ∠BAC = 66° and ∠ABC = 80°.
Calculate:
- ∠DBC,
- ∠IBC,
- ∠BIC.
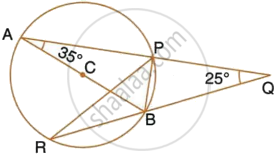
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.

In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.

AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
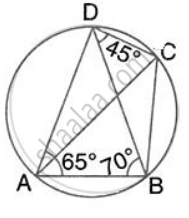
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find: ∠ ACB.
Hence, show that AC is a diameter.
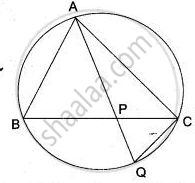
In the given Figure. P is any point on the chord BC of a circle such that AB = AP. Prove that CP = CQ.
In the given figure, ∠CAB = 25°, find ∠BDC, ∠DBA and ∠COB
