Advertisements
Advertisements
प्रश्न
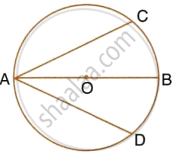
In the given figure, AB is the diameter of a circle with centre O.
If chord AC = chord AD, prove that:
- arc BC = arc DB
- AB is bisector of ∠CAD.
Further, if the length of arc AC is twice the length of arc BC, find:
- ∠BAC
- ∠ABC
उत्तर
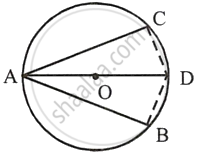
Given – In a circle with centre O, AB is the diameter and AC and AD are two chords such that AC = AD
To prove:
- arc BC = arc DB
- AB is the bisector of ∠CAD
- If arc AC = 2 arc BC, then find
- ∠BAC
- ∠ABC
Construction: Join BC and BD
Proof: In right angled ∆ABC and ∆ABD
Side AC = AD ...[Given]
Hyp. AB = AB ...[Common]
∴ By right Angle – Hypotenuse – Side criterion of congruence
ΔABC ≅ ΔABD
i. The corresponding parts of the congruent triangle are congruent.
∴ BC = BD ...[c.p.c.t]
∴ Arc BC = Arc BD ...[Equal chords have equal arcs]
ii. ∠BAC = ∠BAD
∴ AB is the bisector of ∠CAD
iii. If Arc AC = 2 arc BC,
Then ∠ABC = 2∠BAC
But ∠ABC + ∠BAC = 90°
`=>` 2∠BAC + ∠BAC = 90°
`=>` 3∠BAC = 90°
`=> ∠BAC = (90^circ)/3 = 30^circ`
∠ABC = 2∠BAC
`=>` ∠ABC = 2 × 30° = 60°
APPEARS IN
संबंधित प्रश्न
In the given figure, ∠BAD = 65°, ∠ABD = 70°, ∠BDC = 45°
1) Prove that AC is a diameter of the circle.
2) Find ∠ACB
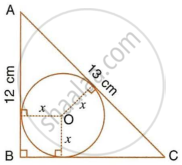
ABC is a right angles triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.
Calculate the value of x, the radius of the inscribed circle.
Prove that the rhombus, inscribed in a circle, is a square.
ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED = 65°; calculate :
- ∠DAB,
- ∠BDC.

Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
Using ruler and a compass only construct a semi-circle with diameter BC = 7cm. Locate a point A on the circumference of the semicircle such that A is equidistant from B and C. Complete the cyclic quadrilateral ABCD, such that D is equidistant from AB and BC. Measure ∠ADC and write it down.
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠ADC
Also, show that the ΔAOD is an equilateral triangle.
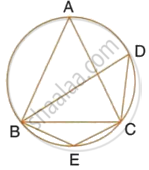
In the figure, ∠DBC = 58°. BD is diameter of the circle.
Calculate:
- ∠BDC
- ∠BEC
- ∠BAC
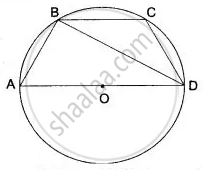
In the figure given alongside, AD is the diameter of the circle. If ∠ BCD = 130°, Calculate: (i) ∠ DAB (ii) ∠ ADB.
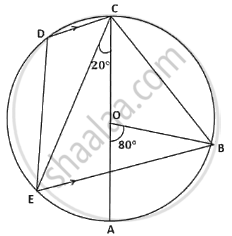
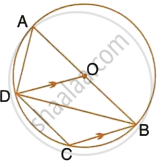
In the given figure AC is the diameter of the circle with centre O. CD is parallel to BE.
∠AOB = 80° and ∠ACE = 20°.
Calculate
- ∠BEC
- ∠BCD
- ∠CED