Advertisements
Advertisements
प्रश्न
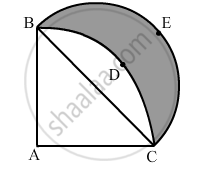
In Figure 5, ABCD is a quadrant of a circle of radius 28 cm and a semi circle BEC is drawn with BC as diameter. Find the area of the shaded region ?\[[Use\pi = \frac{22}{7}]\]
उत्तर
Given:
Radius (r) of the circle = AB = AC = 28 cm
Area of quadrant ABDC:
\[= \frac{1}{4} \times \pi \times r^2 \]
\[ = \left( \frac{1}{4} \times \frac{22}{7} \times 28 \times 28 \right) {cm}^2 \]
\[ = 616 {cm}^2\]
Area of ∆ABC:
\[= \frac{1}{2} \times AC \times AB\]
\[ = \left( \frac{1}{2} \times 28 \times 28 \right) {cm}^2 \]
\[ = 392 {cm}^2\]
Area of segment BDC = Area of quadrant ABDC
= 224 cm2 ....(i)
BC2 = BA2 + AC2 (By Pythagoras theorem)
⇒ BC2 = (282 + 282) cm2
⇒ BC2 =\[28 \times 28 \times 2\] cm2
\[= \frac{1}{2} \times \pi \times r^2 \]
\[ = \left( \frac{1}{2} \times \frac{22}{7} \times 14\sqrt{2} \times 14\sqrt{2} \right) {cm}^2 \]
\[ = \left( \frac{1}{2} \times \frac{22}{7} \times 14 \times 14 \times 2 \right) {cm}^2 \]
\[ = 616 {cm}^2\]
Area of the shaded portion = Area of semi-circle BEC −-Area of segment BDC
= 616 cm2 − 224 cm2
= 392 cm2
APPEARS IN
संबंधित प्रश्न
Find the area of the shaded region in the given figure, if ABCD is a square of side 14 cm and APD and BPC are semicircles. [Use Π = 22/7]

Find the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre [Use Π = 22/7]

In the given figure, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touches externally two of the remaining three circles. Find the area of the shaded region. [Use Π = 22/7]

The radii of two circles are 19cm and 9 cm respectively. Find the radius and area of the circle which has circumferences is equal to sum of circumference of two circles.
Find the area of triangle whose base measures 24 cm and the corresponding height measure 14.5 cm.
Find the area of the triangle whose sides are 18 cm, 24 cm and 30 cm. Also find the height corresponding to the smallest side.
The sides of a triangle are in the ratio 5:12:13 and its perimeter is 150 m. Find the area of the triangle.
Find the perimeter of the shaded region in Figure 4, if ABCD is a square of side 14 cm and APB and CPD are semicircles. Use `pi = 22/7`
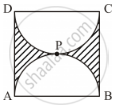
Find the area of the shaded region in figure.
Read the following passage:
Governing council of a local public development authority of Dehradun decided to build an adventurous playground on the top of a hill, which will have adequate space for parking.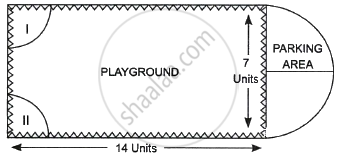 After survey, it was decided to build rectangular playground, with a semi-circular area allotted for parking at one end of the playground. The length and breadth of the rectangular playground are 14 units and 7 units, respectively. There are two quadrants of radius 2 units on one side for special seats. |
Based on the above information, answer the following questions:
- What is the total perimeter of the parking area?
- (a) What is the total area of parking and the two quadrants?
OR
(b) What is the ratio of area of playground to the area of parking area? - Find the cost of fencing the playground and parking area at the rate of ₹ 2 per unit.
