Advertisements
Advertisements
प्रश्न
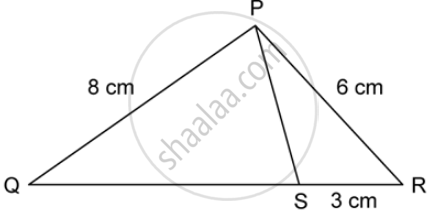
In the adjoining figure ABC is a right angle triangle with ∠BAC = 90°, and AD ⊥ BC.
(i) Prove ΔADB ∼ ΔCDA.
(ii) If BD = 18 cm, CD = 8 cm find AD.
(iii) Find the ratio of the area of ΔADB is to area of ΔCDA.
उत्तर
(i) In ΔADB and ΔADC
AD = AD ...(self)
∠ADC = 90° ...(AD ⊥ BC)
∠ADB = 90° ...(AD ⊥ BC)
then ∠ADC = ∠ADB
so, ΔADB ∼ ΔADC ...(By AAA similarity)
or ΔADB ∼ ΔCDA
Hence proved.
(ii) ∵ ΔADB ∼ ΔCDA
∴ `"AD"/"BD" = "CD"/"AD"`
`("corresponding parts of similar Δ's are proportional")`
or
AD2 = BD x CD
⇒ AD2 = 18 x 8 ...`(("BD" = 18),("CD" = 8),("(given)"))`
⇒ AD2 = 144
AD = 12 cm
(iii) `"Area of ΔADB"/"Area of ΔCDA" = "BD"^2/"AD"^2`
`("Area theorem of similar triangles")`
= `18^2/12^2 = (18 xx 18)/(12 xx 12)`
= `(3 xx 3)/(2 xx 2) = (9)/(4)`
⇒ area (ΔADB) : area (ΔCDA) = 9 : 4.
APPEARS IN
संबंधित प्रश्न
Through the mid-point M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting diagonal AC in L and AD produced in E. Prove that: EL = 2BL.
In the following figure, XY is parallel to BC, AX = 9 cm, XB = 4.5 cm and BC = 18 cm.
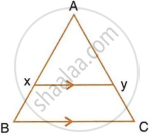
Find : XY
In the following figure, AB, CD and EF are perpendicular to the straight line BDF.
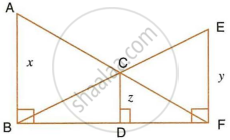
If AB = x and CD = z unit and EF = y unit, prove that : `1/x + 1/y = 1/z`
In the figure given below, AB || EF || CD. If AB = 22.5 cm, EP = 7.5 cm, PC = 15 cm and DC = 27 cm.
Calculate :
- EF
- AC

In the figure given below, AB ‖ EF ‖ CD. If AB = 22.5 cm, EP = 7.5 cm, PC = 15 cm and DC = 27 cm. Calculate : AC
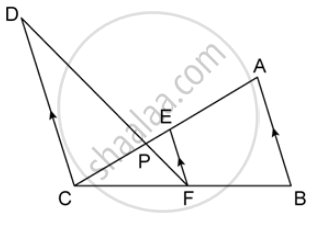
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
- i. ProveΔPQR∼ Δ
- Find the lengths of QR and PS.
- `(Area of DeltaPQR)/(area of Delta SPR)`
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :

ΔADE ~ ΔACB.
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
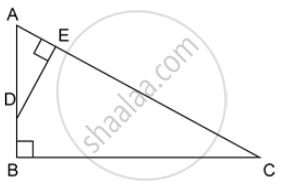
Find, area of ΔADE : area of quadrilateral BCED.
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm2, area (ΔDEF) = 25 cm2 and BC = 2·3 cm find EF.
Triangles ABC and DEF are similar.
If AC = 19 cm and DF = 8 cm, find the ratio between the areas of two triangles.
