Advertisements
Advertisements
प्रश्न
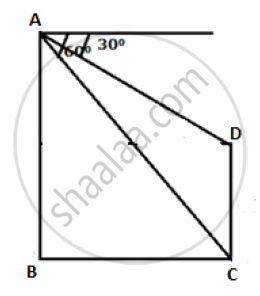
In the figure, given below, it is given that AB is perpandiculer to BD and is of length X metres. DC = 30 m, ∠ADB = 30° and ∠ACB = 45°. Without using tables, find X.

उत्तर
In ΔABC, we have
`tan 45^circ = (AB)/(CB) = X/(CB)` ...`[∵ tan theta = "Perpendicular"/"Base"]`
`1 = X/(CB)`
`\implies` CB = X ...(i)
Again in ΔADB, we have
`tan 30^circ = (AB)/(DB) = X/(DB)`
`\implies 1/sqrt(3) = X/(DB)`
∴ `DB = sqrt(3) xx X` ...(ii)
But DB = DC + CB
∴ `sqrt(3)X = 30 + X`
`\implies sqrt(3)X - X = 30`
`\implies X(sqrt(3) - 1) = 30`
`\implies X = 30/(sqrt(3) - 1)m`
∴ `X = (30(sqrt(3) + 1))/((sqrt(3) - 1)(sqrt(3) + 1))`
= `(30(sqrt(3) + 1))/(3 - 1)m`
= `(30(sqrt(3) + 1))/2`
= 15 (1.732 + 1) m.
= 15 × 2.732
= 40.98 m.
APPEARS IN
संबंधित प्रश्न
In the figure given, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to 30o and 60o respectively. Find:
1) The horizontal distance between AB and CD.
2) The height of the lamp post.
A man observes the angle of elevation of the top of a building to be 30o. He walks towards it in a horizontal line through its base. On covering 60 m the angle of elevation changes to 60o. Find the height of the building correct to the nearest metre.
Prove the following identities:
tan2 A – sin2 A = tan2 A . sin2 A
From the top of a light house 100 m high, the angles of depression of two ships are observed as 48° and 36° respectively. Find the distance between the two ships (in the nearest metre) if:
- the ships are on the same side of the light house,
- the ships are on the opposite sides of the light house.
An aeroplane takes off at angle of `30^circ` with the ground . Find the height of the aeroplane above the ground when it has travelled 386m without changing direction .
The top of a ladder reaches a pcint on the wall 5 m above the ground. If the foot of the ladder makes an angle of 30° with the ground, find the length of the ladder.
Find the angle of depression from the top of a 140m high pillar of a milestone on the ground at a distance of 200m from the foot of the pillar.
The angle of elevation of the top of an unfinished tower at a point 150 m from its base is 30°. How much higher must the tower be raised so that its angle of elevation at the same point may be 60°?
The horizontal distance between two trees of different heights is 100m. The angle of depression of the top of the first tree when seen from the top of the second tree is 45°. If the height of the second tree is 150m, find the height of the first tree.
The angle of elevation of an aeroplane from a point on the ground is 45°. After 15 seconds, the angle of elevation changes to 30°. If the aeroplane is flying at a height of 3000 m, find the speed of the aeroplane.
