Advertisements
Advertisements
प्रश्न
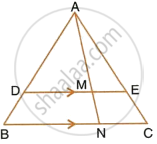
In the given figure, DE || BC, AE = 15 cm, EC = 9 cm, NC = 6 cm and BN = 24 cm.
- Write all possible pairs of similar triangles.
- Find lengths of ME and DM.
उत्तर
i. In ΔAME and ΔANC,
∠AME = ∠ANC ...(Since DE || BC that is, ME || NC)
∠MAE = ∠NAC ...(Common angle)
`=>` ΔAME and ΔANC ...(AA criterion for similarity)
In ΔADM and ΔABN,
∠ADM = ∠ABN ...(Since DE || BC that is, ME || BN)
∠DAM = ∠BAN ...(Common angle)
`=>` ΔADM and ΔABN ...(AA criterion for similarity)
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC that is, ME || NC)
∠AED = ∠ACB ...(Since DE || BC)
`=>` ΔADE and ΔABC ...(AA criterion for similarity)
ii. In ΔAME and ΔANC,
∠AME = ∠ANC ...(Since DE || BC that is, ME || NC)
∠MAE = ∠NAC ...(Common angle)
`=>` ΔAME and ΔANC ...(AA criterion for similarity)
`=> (ME)/(NC) = (AE)/(AC)`
`=> (ME)/6 = 15/24`
`=>` ME = 3.75 cm
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC that is, ME || NC)
∠AED = ∠ACB ...(Since DE || BC)
`=>` ΔADE and ΔABC ...(AA criterion for similarity)
`=> (AD)/(AB) = (AE)/(AC) = 15/24` ...(i)
In ΔADM and ΔABN,
∠ADM = ∠ABN ...(Since DE || BC that is, ME || NC)
∠DAM = ∠BAN ...(Common angle)
`=>` ΔADM and ΔABN ...(AA criterion for similarity)
`=> (DM)/(BN) = (AD)/(AB) = 15/24` ...(From (i))
`=> (DM)/24 = 15/24`
`=>` DM = 15 cm
APPEARS IN
संबंधित प्रश्न
State, true or false:
The diagonals of a trapezium divide each other into proportional segments.
In quadrilateral ABCD, diagonals AC and BD intersect at point E such that
AE : EC = BE : ED. Show that: ABCD is a trapezium.
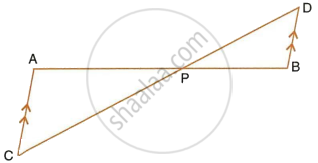
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that: ΔAPC and ΔBPD are similar.
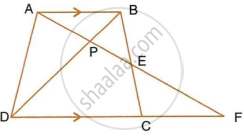
In the following figure, ABCD to a trapezium with AB || DC. If AB = 9 cm, DC = 18 cm, CF = 13.5 cm, AP = 6 cm and BE = 15 cm.
Calculate:
- EC
- AF
- PE
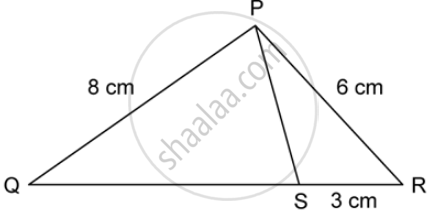
PQR is a triangle. S is a point on the side QR of ΔPQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
- i. ProveΔPQR∼ Δ
- Find the lengths of QR and PS.
- `(Area of DeltaPQR)/(area of Delta SPR)`
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm, area (ΔDEF) = 25 cm2 and BC = 2.3 cm find EF.
Two isosceles triangle have equal vertical angles and their areas are in the ratio of 36 : 25. Find the ratio between their corresponding heights.
In the figure below, PB and QA are perpendiculars to the line segment AB. If PO = 6 cm, QO = 9 cm and the area of ΔPOB = 120 cm2, find the area of ΔQOA.
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm2, area (ΔDEF) = 25 cm2 and BC = 2·3 cm find EF.
Triangles ABC and DEF are similar.
If area (ΔABC) = 36 cm2, area (ΔDEf) = 64 cm2 and DE = 6.2 cm, find AB.
