Advertisements
Advertisements
प्रश्न
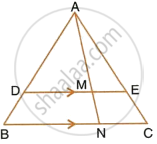
In the given figure, DE || BC, AE = 15 cm, EC = 9 cm, NC = 6 cm and BN = 24 cm. Find lengths of ME and DM.
उत्तर
In ΔAME and ΔANC,
∠AME = ∠ANC ...(Since DE || BC that is, ME || NC)
∠MAE = ∠NAC ...(Common angle)
`=>` ΔAME and ΔANC ...(AA criterion for similarity)
`=> (ME)/(NC) = (AE)/(AC)`
`=> (ME)/6 = 15/24`
`=>` ME = 3.75 cm
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC that is, ME || NC)
∠AED = ∠ACB ...(Since DE || BC)
`=>` ΔADE and ΔABC ...(AA criterion for similarity)
`=> (AD)/(AB) = (AE)/(AC) = 15/24` ...(i)
In ΔADM and ΔABN,
∠ADM = ∠ABN ...(Since DE || BC that is, ME || NC)
∠DAM = ∠BAN ...(Common angle)
`=>` ΔADM and ΔABN ...(AA criterion for similarity)
`=> (DM)/(BN) = (AD)/(AB) = 15/24` ...(From (i))
`=> (DM)/24 = 15/24`
`=>` DM = 15 cm
APPEARS IN
संबंधित प्रश्न
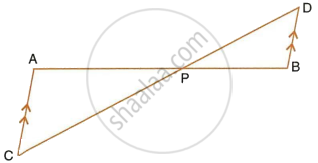
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that: If BD = 2.4 cm, AC = 3.6 cm, PD = 4.0 cm and PB = 3.2 cm; find the lengths of PA and PC.
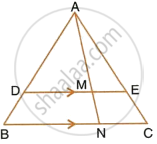
In the given figure, DE || BC, AE = 15 cm, EC = 9 cm, NC = 6 cm and BN = 24 cm.
- Write all possible pairs of similar triangles.
- Find lengths of ME and DM.
Triangle ABC is similar to triangle PQR. If AD and PM are corresponding medians of the two triangles, prove that : `("AB")/("PQ") = ("AD")/("PM")`.
Triangle ABC is similar to triangle PQR. If AD and PM are altitudes of the two triangles, prove that : `(AB)/(PQ) = (AD)/(PM)`.
Triangle ABC is similar to triangle PQR. If bisector of angle BAC meets BC at point D and bisector of angle QPR meets QR at point M, prove that : `(AB)/(PQ) = (AD)/(PM)`.
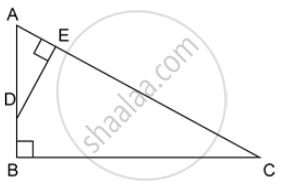
In the given figure, AB and DE are perpendiculars to BC.
Find the ratio of the area of a ΔABC : area of ΔDEC.

ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
Find, area of ΔADE : area of quadrilateral BCED.
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm, area (ΔDEF) = 25 cm2 and BC = 2.3 cm find EF.
Triangles ABC and DEF are similar.
If area (ΔABC) = 36 cm2, area (ΔDEF) = 64 cm2 and DE = 6.2 cm, find AB.
In figure ABC and DBC are two triangles on the same base BC. Prove that
`"Area (ΔABC)"/"Area (ΔDBC)" = "AO"/"DO"`.
