Advertisements
Advertisements
प्रश्न
O(0, 0) is the centre of a circle whose one chord is AB, where the points A and B are (8, 6) and (10, 0) respectively. OD is the perpendicular from the centre to the chord AB. Find the coordinates of the mid-point of OD.
उत्तर
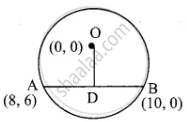
Note: Since OD is perpendicular to AB, OD bisect the chord
D is the mid-point of AB
Mid−point of a line = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
Mid−point of AB(D) = `((8 + 10)/2, (6 + 0)/2)`
= `(18/2, 6/2)`
= (9, 3)
Mid−point of OD = `((0 + 9)/2, (0 + 3)/2)`
= `(9/2, 3/2)`
Mid−point of OD is `(9/2, 3/2)`
APPEARS IN
संबंधित प्रश्न
Points A(–5, x), B(y, 7) and C(1, –3) are collinear (i.e. lie on the same straight line) such that AB = BC. Calculate the values of x and y.
The co-ordinates of the centroid of a triangle PQR are (2, –5). If Q = (–6, 5) and R = (11, 8); calculate the co-ordinates of vertex P.
Find the coordinates of the midpoint of the line segment joining P(0, 6) and Q(12, 20).
A(6, -2), B(3, -2) and C(S, 6) are the three vertices of a parallelogram ABCD. Find the coordinates of the fourth vertex c.
If (-3, 2), (1, -2) and (5, 6) are the midpoints of the sides of a triangle, find the coordinates of the vertices of the triangle.
The coordinates of the end points of the diameter of a circle are (3, 1) and (7, 11). Find the coordinates of the centre of the circle.
A(3, 1), B(y, 4) and C(1, x) are vertices of a triangle ABC. P, Q and R are mid - points of sides BC, CA and AB respectively. Show that the centroid of ΔPQR is the same as the centroid ΔABC.
The three vertices of a parallelogram taken in order are (-1, 0), (3, 1) and (2, 2) respectively. Find the coordinates of the fourth vertex.
The points A(−3, 6), B(0, 7) and C(1, 9) are the mid-points of the sides DE, EF and FD of a triangle DEF. Show that the quadrilateral ABCD is a parallelogram.
The mid-point of the line joining (−a, 2b) and (−3a, −4b) is
