Advertisements
Advertisements
प्रश्न
O is a point in the interior of a square ABCD such that OAB is an equilateral triangle. Show that ∆OCD is an isosceles triangle.
उत्तर
Given: O is a point in the interior of a square ABCD such that ΔOAB is an equilateral triangle.
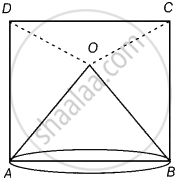
Construction: Join OC and OD.
To show: ΔOCD is an isosceles triangle.
Proof: Since, AOB is an equilateral triangle.
∴ ∠OAB = ∠OBA = 60° ...(i)
Also, ∠DAB = ∠CBA = 90° ...(ii) [Each angle of a square is 90°] [∵ ABCD is a square]
On subtracting equation (i) from equation (ii), we get
∠DAB – ∠OAB = ∠CBA – ∠OBA = 90° – 60°
i.e. ∠DAO = ∠CBO = 30°
In ΔAOD and ΔBOC,
AO = BO ...[Given] [All the side of an equilateral triangle are equal]
∠DAO = ∠CBO ...[Proved above]
And AD = BC ...[Sides of a square are equal]
∴ ΔAOD ≅ ΔBOC ...[By SAS congruence rule]
Hence, OD = OC ...[By CPCT]
In ΔCOD,
OC = OD
Hence, ΔCOD is an isosceles triangle.
Hence proved.
APPEARS IN
संबंधित प्रश्न
If the bisectors of the base angles of a triangle enclose an angle of 135°, prove that the triangle is a right triangle.
Can a triangle have two right angles? Justify your answer in case.
Define a triangle.
An exterior angle of a triangle is equal to 100° and two interior opposite angles are equal. Each of these angles is equal to
Calculate the angles of a triangle if they are in the ratio 4: 5: 6.
One angle of a right-angled triangle is 70°. Find the other acute angle.
The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
7 cm, 24 cm, 25 cm
Find all the three angles of the ΔABC
The exterior angle of a triangle is equal to the sum of two
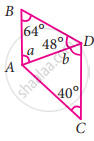
In the given figure, AB is parallel to CD. Then the value of b is