Advertisements
Advertisements
प्रश्न
An exterior angle of a triangle is equal to 100° and two interior opposite angles are equal. Each of these angles is equal to
विकल्प
75°
80°
80°
40°
50°
Advertisements
उत्तर
n the ΔABC, CD is the ray extended from the vertex C of ΔABC. It is given that the exterior angle of the triangle is 100° and two of the interior opposite angles are equal.
So, ∠ACD = 100° and A = ∠B
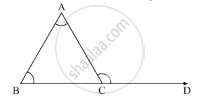
So, now using the property, “an exterior angle of the triangle is equal to the sum of the two opposite interior angles”, we get.
In ΔABC
∠A + ∠B = ∠ACD
∠2A = 100°
`∠A = (100°)/2`
∠A = 50°
∠A = ∠B = 50°
Therefore, each of the two opposite interior angles is 50°.
APPEARS IN
संबंधित प्रश्न
Can a triangle have two acute angles?Justify your answer in case.
In the given figure, AM ⊥ BC and AN is the bisector of ∠A. If ∠B = 65° and ∠C = 33°, find ∠MAN.
In ΔABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 120°, then find the measure of ∠A.
In the given figure, side BC of ΔABC is produced to point D such that bisectors of ∠ABC and ∠ACD meet at a point E. If ∠BAC = 68°, find ∠BEC.
Calculate the unknown marked angles of the following figure :
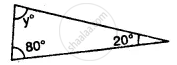
Find the unknown marked angles in the given figure:
The angles of a triangle are in the ratio 2 : 3 : 4. Then the angles are
The image of an object placed at a point A before a plane mirror LM is seen at the point B by an observer at D as shown in the following figure. Prove that the image is as far behind the mirror as the object is in front of the mirror.
[Hint: CN is normal to the mirror. Also, angle of incidence = angle of reflection].
P is a point on the bisector of ∠ABC. If the line through P, parallel to BA meet BC at Q, prove that BPQ is an isosceles triangle.
In the following figure, points lying in the interior of the triangle PQR are ______, that in the exterior are ______ and that on the triangle itself are ______.
