Advertisements
Advertisements
प्रश्न
Answer the following question.
Obtain the expression for the energy stored in a capacitor connected across a dc battery. Hence define energy density of the capacitor
उत्तर
Energy Stored in a Charged Capacitor:
The energy of a charged capacitor is measured by the total work done in charging the capacitor to a given potential.
Let us assume that initially both the plates are uncharged. Now, we have to repeatedly remove small positive charges from one plate and transfer them to the other plate.
Let
q →Total quantity of charge transferred
V →Potential difference between the two plates
Then,
q = CV
Now, when an additional small charge dq is transferred from the negative plate to the positive plate, the small work done is given by,
`dW = Vdq = q/"C" dq`
The total work done in transferring charge Q is given by,
`W = int_0^Q q/"C"dq = 1/"C"int_0^Qqdq = 1/"C"[q^2/2]_0^Q`
`W = Q^2/(2"C")`
This work done is stored as electrostatic potential energy U in the capacitor.
`U = Q^2/(2"C")`
Hence energy stored in the capacitor = `1/2 Q^2/"C" = (Asigma)^2/2 xx d/(epsilon_oA)`
The surface charge density `sigma` is related to the electric field `E` between the plates, `E` = `sigma/epsilon_o`
So, energy stored in the capacitor = `1/2epsilon_oE^2 xx Ad`
Here, Ad is volume between the plates of capacitor.
We define energy density as energy stored per unit volume of space.
Energy density of electric field = `U = 1/2epsilon_oE^2`
APPEARS IN
संबंधित प्रश्न
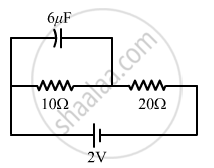
Find the charge on the capacitor as shown in the circuit.
The energy density in the electric field created by a point charge falls off with the distance from the point charge as
A capacitor C1 of capacitance 1 μF and a capacitor C2 of capacitance 2 μF are separately charged by a common battery for a long time. The two capacitors are then separately discharged through equal resistors. Both the discharge circuits are connected at t = 0.
(a) The current in each of the two discharging circuits is zero at t = 0.
(b) The currents in the two discharging circuits at t = 0 are equal but not zero.
(c) The currents in the two discharging circuits at t = 0 are unequal.
(d) C1 loses 50% of its initial charge sooner than C2 loses 50% of its initial charge.
How many time constants will elapse before the energy stored in the capacitor reaches half of its equilibrium value in a charging RC circuit?
A capacitor of capacitance 12.0 μF is connected to a battery of emf 6.00 V and internal resistance 1.00 Ω through resistanceless leads. 12.0 μs after the connections are made, what will be (a) the current in the circuit (b) the power delivered by the battery (c) the power dissipated in heat and (d) the rate at which the energy stored in the capacitor is increasing?
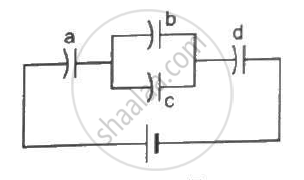
Each capacitor in figure has a capacitance of 10 µF. The emf of the battery is 100 V. Find the energy stored in each of the four capacitors.
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an uncharged capacitor of equal capacitance through a resistance R. Find the charge on the second capacitor as a function of time.
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an ideal battery of emf ε through a resistance R. Find the charge on the capacitor at time t.
Do free electrons travel to region of higher potential or lower potential?
Derive an expression for energy stored in a capacitor.
