Advertisements
Advertisements
प्रश्न
One side of a rectangle is 12 cm long and its diagonal measure 37 cm. Find the other side and the area of the rectangle.
उत्तर
One side of the rectangle = 12 cm
Diagonal of the rectangle = 37 cm
The diagonal of a rectangle forms the hypotenuse of a right-angled triangle. The other two sides of the triangle are the length and the breadth of the rectangle.
Now, using Pythagoras’ theorem, we have:
`"(One side)"^2+"(Other side)"^2="(hypotenuse)"^2`
⇒`(12)^2+"(other side)"^2=(37)^2`
⇒`144+(Other side)^2=1369`
⇒`(Other side)^2=1329-144`
⇒`(Other side)^2=1225`
⇒`(Other side)=sqrt1225`
⇒`Other side=35 cm`
Thus, we have:
Length = 35 cm
Breadth = 12 cm
Area of the rectangle=`35xx12=420 cm^2`
APPEARS IN
संबंधित प्रश्न
A chord of a circle of radius 20 cm sub tends an angle of 900 at the centre . Find the area of the corresponding major segment of the circle
( Use \[\pi = 3 . 14\])
A rectangular park is 100 m by 50 m. It is surrounding by semi-circular flower beds all round. Find the cost of levelling the semi-circular flower beds at 60 paise per square metre (use π = 3.14).
The ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal, is
Area of the largest triangle that can be inscribed in a semi-circle of radius r units is ______.
Find the lengths of the arcs cut off from a circle of radius 12 cm by a chord 12 cm long. Also, find the area of the minor segment.
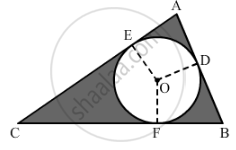
In the given figure, ∆ABC is right-angled at A. Find the area of the shaded region if AB = 6 cm, BC = 10 cm and O is the centre of the incircle of ∆ABC.
The minute hand of a clock is 12 cm long. Find the area swept by in it 35 minutes.
The radii of the inner and outer circumferences of a circular running track are 63 m and 70 m respectively. Find :
(i) the area of the track ;
(ii) the difference between the lengths of the two circumferences of the track.
A circular park is surrounded by a road 21 m wide. If the radius of the park is 105 m, find the area of the road.
Find the area of the shaded region: