Advertisements
Advertisements
प्रश्न
Points P and Q trisect the line segment joining the points A(−2, 0) and B(0, 8) such that P is near to A. Find the coordinates of points P and Q.
उत्तर
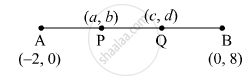
P and Q trisect line joining the points A and B.
Let the coordinates of P and Q be (a, b) and (c, d) respectively.
P is the midpoint of AQ.
`(-2 +"c")/2 = "a" and (0+"d")/2 = "b"`
⇒ c= 2a + 2 and d = 2b ...........(1)
Also, Q is the mid point of PB.
`("a"+0)/2 ="c" and ("b"+8)/2 = "d"`
⇒ `"a"/2 = "c" and ("b"+8)/2 ="d"` .........(2)
From (1) and (2) we have
2a + 2 =`"a"/2`
⇒ 4a + 4 = a
⇒ 3a = -4
⇒`"a" = -4/3`
Also,
2b `=("b"+8)/2`
⇒ 4b = b +8
⇒ b = `8/3`
Putting these values of a and b in (2)
`((-4)/3)/2 = "c"`
⇒ `(-2)/3 = "c"`
And
`(8/3+8)/2 = "d"`
`((8+24)/3)/2 = "d"`
⇒`(32/2)/2 = "d"`
⇒ `16/3 = "d"`
Thus, the points are P `((-4)/3 , 8/3)` and Q `((-2)/3 , 16/3).`
APPEARS IN
संबंधित प्रश्न
Find the ratio in which the line segment joining the points A(3,- 3) and B(- 2, 7) is divided by x-axis. Also find the coordinates of the point of division.
Construct an isosceles triangle with base 8 cm and altitude 4 cm. Construct another triangle whose sides are `2/3` times the corresponding sides of the isosceles triangle.
Construct a triangle similar to a given ΔXYZ with its sides equal to (3/4)th of the corresponding sides of ΔXYZ. Write the steps of construction.
Construct a right triangle in which the sides, (other than the hypotenuse) are of length 6 cm and 8 cm. Then construct another triangle, whose sides are `3/5` times the corresponding sides of the given triangle.
Choose the correct alternative:
______ number of tangents can be drawn to a circle from the point on the circle.
If the point P (6, 7) divides the segment joining A(8, 9) and B(1, 2) in some ratio, find that ratio
Solution:
Point P divides segment AB in the ratio m: n.
A(8, 9) = (x1, y1), B(1, 2 ) = (x2, y2) and P(6, 7) = (x, y)
Using Section formula of internal division,
∴ 7 = `("m"(square) - "n"(9))/("m" + "n")`
∴ 7m + 7n = `square` + 9n
∴ 7m – `square` = 9n – `square`
∴ `square` = 2n
∴ `"m"/"n" = square`
For ∆ABC in which BC = 7.5cm, ∠B =45° and AB - AC = 4, select the correct figure.
Match the following based on the construction of similar triangles, if scale factor `(m/n)` is.
| Column I | Column II | ||
| i | >1 | a) | The similar triangle is smaller than the original triangle. |
| ii | <1 | b) | The two triangles are congruent triangles. |
| iii | =1 | c) | The similar triangle is larger than the original triangle. |
What is the ratio `(AC)/(BC)` for the line segment AB following the construction method below?
Step 1: A ray is extended from A and 30 arcs of equal lengths are cut, cutting the ray at A1, A2,…A30
Step 2: A line is drawn from A30 to B and a line parallel to A30B is drawn, passing through the point A17 and meet AB at C.
Two line segments AB and AC include an angle of 60° where AB = 5 cm and AC = 7 cm. Locate points P and Q on AB and AC, respectively such that AP = `3/4` AB and AQ = `1/4` AC. Join P and Q and measure the length PQ.
