Advertisements
Advertisements
प्रश्न
Prove that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
उत्तर
To prove: In a right-angled triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides of the triangle.
Proof: Let PQR be a triangle, right-angled at P.
Draw PS ⊥ QR
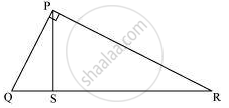
Now, we know that if a perpendicular is drawn from the vertex of the right angle of a right triangle to the hypotenuse, then the triangles on both sides of the perpendicular are similar to the whole triangle and to each other.
∴ΔQSP ∼ ΔQPR
Therefore, `"QS"/"QP" = "QP"/"QR"` .....................(Since the sides of similar triangles are proportional)
⇒ QS. QR = Qp2 .......(1)
Also, we have
ΔPSR ∼ ΔQPR
Therefore, `"RS"/"RP" = "RP"/"RQ"`.............(Since the sides of similar triangles are proportional)
⇒ RS.RQ = RP2 … (2)
Adding equations (1) and (2), we obtain
QS.QR + RS.RQ = RP2 + QP2
⇒ QR. (QS + RS) = RP2 + QP2
⇒ QR.QR = RP2 + QP2
⇒ QR2 = RP2 + QP2
Thus, the square of the hypotenuse is equal to the sum of squares of the other two sides of the triangle.
APPEARS IN
संबंधित प्रश्न
In two similar triangles ABC and PQR, if their corresponding altitudes AD and PS are in the ratio 4 : 9, find the ratio of the areas of ∆ABC and ∆PQR
D, E, F are the mid-point of the sides BC, CA and AB respectively of a ∆ABC. Determine the ratio of the areas of ∆DEF and ∆ABC.
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
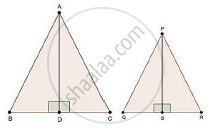
The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
Two isosceles triangles have equal vertical angles and their areas are in the ratio 36 : 25. Find the ratio of their corresponding heights.
The corresponding altitudes of two similar triangles are 6 cm and 9 cm respectively. Find the ratio of their areas.
If ΔABC and ΔBDE are equilateral triangles, where D is the mid-point of BC, find the ratio of areas of ΔABC and ΔBDE.
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
In a rectangle Length = 8 cm, Breadth = 6 cm. Then its diagonal = ______.
Find the length of ST, if ΔPQR ∼ ΔPST.

