Advertisements
Advertisements
प्रश्न
Prove that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
Advertisements
उत्तर
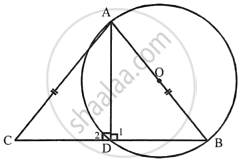
Given – In ∆ABC, AB = AC and a circle with AB as diameter is drawn
Which intersects the side BC and D.
To prove – D is the mid point of BC
Construction – Join AD.
Proof – ∠1 = 90° ...[Angle in a semi circle]
But ∠1 + ∠2 = 180° ...[Linear pair]
∴ ∠2 = 90°
Now in right ∆ABD and ∆ACD,
Hyp. AB = Hyp. AC ...[Given]
Side AD = AD ...[Common]
∴ By the right Angle – Hypotenuse – side criterion of congruence, we have
ΔABD ≅ ∆ACD ...[RHS criterion of congruence]
The corresponding parts of the congruent triangle are congruent.
∴ BD = DC ...[c.p.c.t]
Hence D is the mid point of BC.
संबंधित प्रश्न
In the figure, given alongside, AB || CD and O is the centre of the circle. If ∠ADC = 25°; find the angle AEB. Give reasons in support of your answer.
ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED = 65°, calculate:
- ∠DAB,
- ∠BDC.
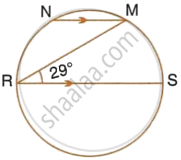
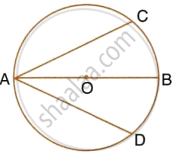
In the given figure, AB is a diameter of the circle. Chord ED is parallel to AB and ∠EAB = 63°.
Calculate:
- ∠EBA,
- ∠BCD.
In the following figure, AD is the diameter of the circle with centre O. Chords AB, BC and CD are equal. If ∠DEF = 110°, calculate: ∠AEF
Using ruler and a compass only construct a semi-circle with diameter BC = 7cm. Locate a point A on the circumference of the semicircle such that A is equidistant from B and C. Complete the cyclic quadrilateral ABCD, such that D is equidistant from AB and BC. Measure ∠ADC and write it down.
In the following figure, AD is the diameter of the circle with centre O. chords AB, BC and CD are equal. If ∠DEF = 110°, Calculate: ∠FAB.
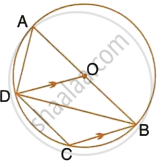
In the given figure, O is the centre of the circle and ∠PBA = 45°. Calculate the value of ∠PQB.
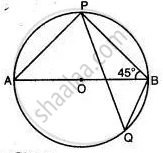
In the given figure, BAD = 65°, ABD = 70°, BDC = 45°.
(i) Prove that AC is a diameter of the circle.
(ii) Find ACB.
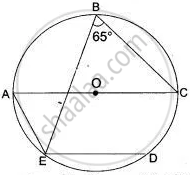
In the figure, ∠DBC = 58°. BD is diameter of the circle.
Calculate:
- ∠BDC
- ∠BEC
- ∠BAC
