Advertisements
Advertisements
प्रश्न
Show that ΔABC, where A(–2, 0), B(2, 0), C(0, 2) and ΔPQR where P(–4, 0), Q(4, 0), R(0, 2) are similar triangles.
उत्तर
In ΔABC, the coordinates of the vertices are A(–2, 0), B(2, 0), C(0, 2).
\[AB = \sqrt{\left( 2 + 2 \right)^2 + \left( 0 - 0 \right)^2} = 4\]
\[BC = \sqrt{\left( 0 - 2 \right)^2 + \left( 2 - 0 \right)^2} = \sqrt{8} = 2\sqrt{2}\]
\[CA = \sqrt{\left( 0 + 2 \right)^2 + \left( 2 - 0 \right)^2} = \sqrt{8} = 2\sqrt{2}\]
In ΔPQR, the coordinates of the vertices are P(–4, 0), Q(4, 0), R(0, 4).
\[PQ = \sqrt{\left( 4 + 4 \right)^2 + \left( 0 - 0 \right)^2} = 8\]
\[QR = \sqrt{\left( 0 - 4 \right)^2 + \left( 4 - 0 \right)^2} = 4\sqrt{2}\]
\[PR = \sqrt{\left( 0 + 4 \right)^2 + \left( 4 - 0 \right)^2} = 4\sqrt{2}\]
Now, for ΔABC and ΔPQR to be similar, the corresponding sides should be proportional.
\[So, \frac{AB}{PQ} = \frac{BC}{QR} = \frac{CA}{PR}\]
\[ \Rightarrow \frac{4}{8} = \frac{2\sqrt{2}}{4\sqrt{2}} = \frac{2\sqrt{2}}{4\sqrt{2}} = \frac{1}{2}\]
Thus, ΔABC is similar to ΔPQR.
APPEARS IN
संबंधित प्रश्न
Find the third vertex of a triangle, if two of its vertices are at (−3, 1) and (0, −2) and the centroid is at the origin.
Prove that the points (0, 0), (5, 5) and (-5, 5) are the vertices of a right isosceles triangle.
Find the equation of the perpendicular bisector of the line segment joining points (7, 1) and (3,5).
Find the ratio in which the line segment joining (-2, -3) and (5, 6) is divided by y-axis. Also, find the coordinates of the point of division in each case.
Show that the points A(2,1), B(5,2), C(6,4) and D(3,3) are the angular points of a parallelogram. Is this figure a rectangle?
Find the coordinates of the midpoints of the line segment joining
A(3,0) and B(-5, 4)
ABCD is rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). If P,Q,R and S be the midpoints of AB, BC, CD and DA respectively, Show that PQRS is a rhombus.
Find the area of a quadrilateral ABCD whose vertices area A(3, -1), B(9, -5) C(14, 0) and D(9, 19).
Find the area of quadrilateral PQRS whose vertices are P(-5, -3), Q(-4,-6),R(2, -3) and S(1,2).
Points (−4, 0) and (7, 0) lie
The ordinate of any point on x-axis is
A point whose abscissa is −3 and ordinate 2 lies in
If A(−3, 5), B(−2, −7), C(1, −8) and D(6, 3) are the vertices of a quadrilateral ABCD, find its area.
If P (x, 6) is the mid-point of the line segment joining A (6, 5) and B (4, y), find y.
If (x , 2), (−3, −4) and (7, −5) are collinear, then x =
A line intersects the y-axis and x-axis at P and Q , respectively. If (2,-5) is the mid-point of PQ, then the coordinates of P and Q are, respectively
What is the form of co-ordinates of a point on the X-axis?
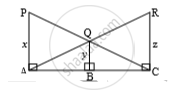
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Seg AB is parallel to X-axis and coordinates of the point A are (1, 3), then the coordinates of the point B can be ______.
