Advertisements
Advertisements
प्रश्न
Show that the points A(2,1), B(5,2), C(6,4) and D(3,3) are the angular points of a parallelogram. Is this figure a rectangle?
उत्तर
The given points are s A(2,1), B(5,2), C(6,4) and D(3,3)
`AB = sqrt((5-2)^2 +(2-1)^2 ) = sqrt((3)^2 +(1)^2 ) = sqrt(9+1) = sqrt(10) ` units
`BC = sqrt((6-5)^2 +(4-2)^2 )= sqrt((1)^2 +(2)^3) = sqrt(1+4) = sqrt(5) `units
`CD = sqrt((3-6)^2 +(3-4)^2) = sqrt((-3)^2 +(-1)^2) = sqrt(9+1) = sqrt(10) `units
`AD = sqrt((3-2)^2+(3-1)^2) = sqrt((1)^2 +(2)^2) = sqrt(1+4) = sqrt(5) ` units
Thus, AB = CD = `sqrt(10) "units and " BC= AD = sqrt(5) ` units
So, quadrilateral ABCD is a parallelogram
`Also , AC = sqrt((6-2)^2 +(4-1)^2) = sqrt((4)^2 +(3)^2 )= sqrt(16+9) = sqrt(25) = 5 ` units
`BD = sqrt((3-5) ^2 +(3-2)^2 ) = sqrt((-2)^2 +(1)^2) = sqrt(4+1) = sqrt(5) units `
But diagonal AC is not equal to diagonal BD. Hence, the given points do not form a rectangle.
APPEARS IN
संबंधित प्रश्न
Find the distance between the following pair of points:
(a, 0) and (0, b)
If the point A (4,3) and B ( x,5) lies on a circle with the centre o (2,3) . Find the value of x.
Show that the following points are the vertices of a square:
A (6,2), B(2,1), C(1,5) and D(5,6)
Point A lies on the line segment PQ joining P(6, -6) and Q(-4, -1) in such a way that `(PA)/( PQ)=2/5` . If that point A also lies on the line 3x + k( y + 1 ) = 0, find the value of k.
The midpoint of the line segment joining A (2a, 4) and B (-2, 3b) is C (1, 2a+1). Find the values of a and b.
`"Find the ratio in which the poin "p (3/4 , 5/12) " divides the line segment joining the points "A (1/2,3/2) and B (2,-5).`
Find the coordinates of the points of trisection of the line segment joining the points (3, –2) and (–3, –4) ?
Show that A(-4, -7), B(-1, 2), C(8, 5) and D(5, -4) are the vertices of a
rhombus ABCD.
Find the area of a parallelogram ABCD if three of its vertices are A(2, 4), B(2 + \[\sqrt{3}\] , 5) and C(2, 6).
If the points A(−1, −4), B(b, c) and C(5, −1) are collinear and 2b + c = 4, find the values of b and c.
Find the coordinates of the point which is equidistant from the three vertices A (\[2x, 0) O (0, 0) \text{ and } B(0, 2y) of ∆\] AOB .
If (−1, 2), (2, −1) and (3, 1) are any three vertices of a parallelogram, then
Any point on the line y = x is of the form ______.
What is the nature of the line which includes the points (-5, 5), (6, 5), (-3, 5), (0, 5)?
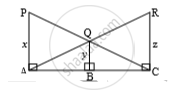
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Find the point on the y-axis which is equidistant from the points (5, −2) and (−3, 2).
Point P(– 4, 2) lies on the line segment joining the points A(– 4, 6) and B(– 4, – 6).
Ordinate of all points on the x-axis is ______.
The point at which the two coordinate axes meet is called the ______.
