Advertisements
Advertisements
प्रश्न
Show that the points (2, 0), (– 2, 0) and (0, 2) are vertices of a triangle. State the type of triangle with reason
उत्तर
Let the points be P(2, 0), Q(– 2, 0) and R(0, 2)
Distance between two points = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
By distance formula,
d(P, Q) = `sqrt([(-2) - 2]^2 + (0 - 0)^2`
= `sqrt((-4)^2 + (0)^2`
= `sqrt(16 + 0)`
= 4 .....(i)
d(Q, R) = `sqrt([0 - (-2)]^2 + (2 - 0)^2`
= `sqrt((2)^2 + (2)^2`
= `sqrt(4 + 4)`
= `sqrt(8)` ......(ii)
d (P, R) = `sqrt((0 -2)^2 + (2 - 0)^2`
= `sqrt((- 2)^2 + (2)^2`
= `sqrt(4 + 4)`
= `sqrt(8)` ......(iii)
On adding (ii) and (iii),
d(P, Q) + d(Q, R) = `4 + sqrt(8)`
`4 + sqrt(8) > sqrt(8)`
∴ d(P, Q) + d(Q, R) > d(P, R)
∴ Points P, Q, R are non colinear points.
We can construct a triangle through 3 non collinear points.
∴ The segment joining the given points form a triangle.
Since P(Q, R) = P(P, R)
∴ ∆PQR is an isosceles triangle.
∴ The segment joining the points (2, 0), (– 2, 0) and (0, 2) will form an isosceles triangle.
APPEARS IN
संबंधित प्रश्न
If the point (x, y) is equidistant from the points (a + b, b – a) and (a – b, a + b), prove that bx = ay
In a classroom, 4 friends are seated at the points A, B, C and D as shown in the following figure. Champa and Chameli walk into the class and after observing for a few minutes, Champa asks Chameli, “Don’t you think ABCD is a square?” Chameli disagrees.
Using distance formula, find which of them is correct.

Name the type of quadrilateral formed, if any, by the following point, and give reasons for your answer:
(4, 5), (7, 6), (4, 3), (1, 2)
Find the point on the x-axis which is equidistant from (2, -5) and (-2, 9).
Find the distance between the points
A(-6,-4) and B(9,-12)
If P (x , y ) is equidistant from the points A (7,1) and B (3,5) find the relation between x and y
Find the distance between the following pair of point.
T(–3, 6), R(9, –10)
Find the relation between a and b if the point P(a ,b) is equidistant from A (6,-1) and B (5 , 8).
Find the coordinates of O, the centre passing through A( -2, -3), B(-1, 0) and C(7, 6). Also, find its radius.
Find the point on y-axis whose distances from the points A (6, 7) and B (4, -3) are in the ratio 1: 2.
Show that the quadrilateral with vertices (3, 2), (0, 5), (- 3, 2) and (0, -1) is a square.
The distance between points P(–1, 1) and Q(5, –7) is ______
Find distance between point A(–1, 1) and point B(5, –7):
Solution: Suppose A(x1, y1) and B(x2, y2)
x1 = –1, y1 = 1 and x2 = 5, y2 = – 7
Using distance formula,
d(A, B) = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
∴ d(A, B) = `sqrt(square +[(-7) + square]^2`
∴ d(A, B) = `sqrt(square)`
∴ d(A, B) = `square`
Show that A(1, 2), (1, 6), C(1 + 2 `sqrt(3)`, 4) are vertices of a equilateral triangle
Seg OA is the radius of a circle with centre O. The coordinates of point A is (0, 2) then decide whether the point B(1, 2) is on the circle?
Using distance formula decide whether the points (4, 3), (5, 1), and (1, 9) are collinear or not.
The coordinates of the point which is equidistant from the three vertices of the ΔAOB as shown in the figure is ______.

Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
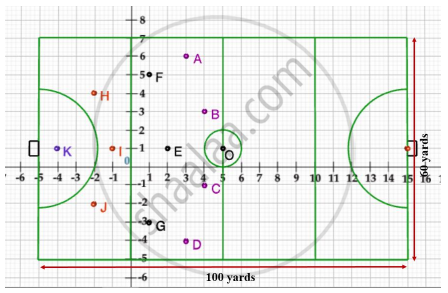
The point on y axis equidistant from B and C is ______.
Find a point which is equidistant from the points A(–5, 4) and B(–1, 6)? How many such points are there?
|
In a GPS, The lines that run east-west are known as lines of latitude, and the lines running north-south are known as lines of longitude. The latitude and the longitude of a place are its coordinates and the distance formula is used to find the distance between two places. The distance between two parallel lines is approximately 150 km. A family from Uttar Pradesh planned a round trip from Lucknow (L) to Puri (P) via Bhuj (B) and Nashik (N) as shown in the given figure below.
|
Based on the above information answer the following questions using the coordinate geometry.
- Find the distance between Lucknow (L) to Bhuj (B).
- If Kota (K), internally divide the line segment joining Lucknow (L) to Bhuj (B) into 3 : 2 then find the coordinate of Kota (K).
- Name the type of triangle formed by the places Lucknow (L), Nashik (N) and Puri (P)
[OR]
Find a place (point) on the longitude (y-axis) which is equidistant from the points Lucknow (L) and Puri (P).

