Advertisements
Advertisements
प्रश्न
Side AC of a ABC is produced to point E so that CE = `(1)/(2)"AC"`. D is the mid-point of BC and ED produced meets AB at F. Lines through D and C are drawn parallel to AB which meets AC at point P and EF at point R respectively. Prove that: 3DF = EF
उत्तर
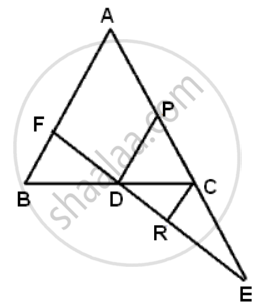
In ΔBDF and ΔDRC,
BD = DC ...(D is the mid-point of BC)
CR || PD || AB
∠BFD = DRC ...(alternate angles)
∠BDF = RDC ...(vertivally opposite angles)
Therefore,
ΔBDF ≅ ΔDRC
⇒ DF = DR .....(i)
In ΔABC,
D is the mid-point of BC and DP || AB
Therefore, P is the mid-point of AC.
In ΔDEP,
C is the mid-point of PE and DP || RC || AB ...(CE = `(1)/(2)"AC"` and P is the mid-point of AC)
Therefore, R is the mid-point of DE.
⇒ DR = RE ......(ii)
But EF = DF + DR + RE
EF = DF + DF + DF
EF = 3DF.
APPEARS IN
संबंधित प्रश्न
In a triangle ∠ABC, ∠A = 50°, ∠B = 60° and ∠C = 70°. Find the measures of the angles of
the triangle formed by joining the mid-points of the sides of this triangle.
In a ΔABC, BM and CN are perpendiculars from B and C respectively on any line passing
through A. If L is the mid-point of BC, prove that ML = NL.
In the given figure, seg PD is a median of ΔPQR. Point T is the mid point of seg PD. Produced QT intersects PR at M. Show that `"PM"/"PR" = 1/3`.
[Hint: DN || QM]

In triangle ABC, AD is the median and DE, drawn parallel to side BA, meets AC at point E.
Show that BE is also a median.
In triangle ABC ; D and E are mid-points of the sides AB and AC respectively. Through E, a straight line is drawn parallel to AB to meet BC at F.
Prove that BDEF is a parallelogram. If AB = 16 cm, AC = 12 cm and BC = 18 cm,
find the perimeter of the parallelogram BDEF.
In ΔABC, AB = 12 cm and AC = 9 cm. If M is the mid-point of AB and a straight line through M parallel to AC cuts BC in N, what is the length of MN?
Prove that the straight lines joining the mid-points of the opposite sides of a quadrilateral bisect each other.
AD is a median of side BC of ABC. E is the midpoint of AD. BE is joined and produced to meet AC at F. Prove that AF: AC = 1 : 3.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if ______.
The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is ______.
