Advertisements
Advertisements
प्रश्न
The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is ______.
विकल्प
a rhombus
a rectangle
a square
any parallelogram
उत्तर
The figure obtained by joining the mid-points of the sides of a rhombus, taken in order, is a rectangle.
Explanation:
The Midpoint Theorem states that the segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side.

Join AC, RP and SQ
In ∆ABC,
P is midpoint of AB and Q is midpoint of BC
∴ By midpoint theorem,
PQ || AC and PQ = `1/2` AC ...(1)
Similarly,
In ∆DAC,
S is midpoint of AD and R is midpoint of CD
∴ By midpoint theorem,
SR || AC and SR = `1/2` AC ...(2)
From (1) and (2),
PQ || SR and PQ = SR
⇒ PQRS is a parallelogram
ABQS is a parallelogram
⇒ AB = SQ
PBCR is a parallelogram
⇒ BC = PR
⇒ AB = PR ...[∵ BC = AB, sides of rhombus]
⇒ SQ = PR
∴ Diagonals of the parallelogram are equal.
Hence, it is a rectangle.
APPEARS IN
संबंधित प्रश्न
ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
In Fig. below, M, N and P are the mid-points of AB, AC and BC respectively. If MN = 3 cm, NP = 3.5 cm and MP = 2.5 cm, calculate BC, AB and AC.
In the given figure, points X, Y, Z are the midpoints of side AB, side BC and side AC of ΔABC respectively. AB = 5 cm, AC = 9 cm and BC = 11 cm. Find the length of XY, YZ, XZ.

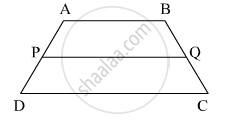
In the Figure, `square`ABCD is a trapezium. AB || DC. Points P and Q are midpoints of seg AD and seg BC respectively. Then prove that, PQ || AB and PQ = `1/2 ("AB" + "DC")`.
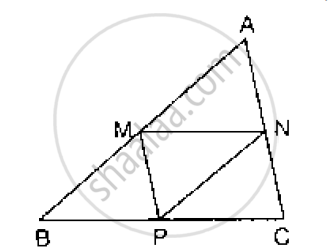
In triangle ABC, M is mid-point of AB and a straight line through M and parallel to BC cuts AC in N. Find the lengths of AN and MN if Bc = 7 cm and Ac = 5 cm.
The figure, given below, shows a trapezium ABCD. M and N are the mid-point of the non-parallel sides AD and BC respectively. Find:

- MN, if AB = 11 cm and DC = 8 cm.
- AB, if DC = 20 cm and MN = 27 cm.
- DC, if MN = 15 cm and AB = 23 cm.
In triangle ABC; M is mid-point of AB, N is mid-point of AC and D is any point in base BC. Use the intercept Theorem to show that MN bisects AD.
The diagonals of a quadrilateral intersect each other at right angle. Prove that the figure obtained by joining the mid-points of the adjacent sides of the quadrilateral is a rectangle.
The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if ______.
E and F are respectively the mid-points of the non-parallel sides AD and BC of a trapezium ABCD. Prove that EF || AB and EF = `1/2` (AB + CD).
[Hint: Join BE and produce it to meet CD produced at G.]
