Advertisements
Advertisements
प्रश्न
Solve the following pair of equations:
`x/a + y/b = a + b, x/a^2 + y/b^2 = 2, a, b ≠ 0`
Advertisements
उत्तर
Given pair of linear equations is
`x/a + y/b` = a + b .....(i)
And `x/a^2 + y/b^2` = 2, a, b ≠ 0 ......(ii)
On multiplying equation (i) by `1/a` and then subtracting from equation (ii), we get
`x/a^2 + y/b^2 = 2`
`x/a^2 + y/(ab) = 1 + b/a`
– – –
`y(1/b^2 - 1/(ab)) = 2 - 1 - b/a`
⇒ `y((a - b)/(ab^2)) = 1 - b/a = ((a - b)/a)`
⇒ y = `(ab^2)/a`
⇒ y = b2
Now, put the value of y in equation (ii), we get
`x/a^2 + b^2/b^2` = 2
⇒ `x/a^2` = 2 – 1 = 1
⇒ x = a2
Hence, the required values of x and y are a2 and b2, respectively.
APPEARS IN
संबंधित प्रश्न
A train travels at a certain average speed for a distance of 54 km and then travels a distance of 63 km at an average speed of 6 km/h more than the first speed. If it takes 3 hours to complete the total journey, what is its first speed?
A thief runs with a uniform speed of 100 m/minute. After one minute, a policeman runs after the thief to catch him. He goes with a speed of 100 m/minute in the first minute and increases his speed by 10 m/minute every succeeding minute. After how many minutes the policeman will catch the thief.
A thief, after committing a theft, runs at a uniform speed of 50 m/minute. After 2 minutes, a policeman runs to catch him. He goes 60 m in first minute and increases his speed by 5 m/minute every succeeding minute. After how many minutes, the policeman will catch the thief?
Solve the following system of equations by cross-multiplication method x + y = a – b; ax – by = a2 + b2
For which value of k will the following pair of linear equations have no solution?
3x + y = 1
(2k – 1)x + (k – 1)y = 2k + 1
Form the pair of linear equations in the following problems and find their solutions (if they exist) by any algebraic method:
A fraction becomes `1/3` when 1 is subtracted from the numerator and it becomes `1/4` when 8 is added to its denominator. Find the fraction.
Solve the following system of equations:
`sqrt(2)x - sqrt(3)y = 0`
`sqrt(3)x - sqrt(8)y = 0`
Solve the following system of equations:
0.5x + 0.7y = 0.74
0.3x + 0.5y = 0.5
Solve the following system of equations by the method of cross-multiplication:
ax + by = a2
bx + ay = b2
Solve each of the following systems of equations by the method of cross-multiplication
`x/a + y/b = 2`
`ax - by = a^2 - b^2`
Solve each of the following systems of equations by the method of cross-multiplication
`x/a + y/b = a + b`
Solve each of the following systems of equations by the method of cross-multiplication :
`2/x + 3/y = 13`
`5/4 - 4/y = -2`
where `x != 0 and y != 0`
Solve the following system of equations by the method of cross-multiplication:
(a + 2b)x + (2a – b)y = 2
(a – 2b)x + (2a + b)y = 3
Solve the following system of equations by the method of cross-multiplication:
`57/(x + y) + 6/(x - y) = 5`
`38/(x + y) + 21/(x - y) = 9`
Solve the system of equations by using the method of cross multiplication:
x + 2y + 1 = 0, 2x – 3y – 12 = 0
A father’s age is three times the sum of the ages of his two children. After 5 years his age will be two times the sum of their ages. Find the present age of the father.
Complete the activity to find the value of x.
3x + 2y = 11 ...(i) and 2x + 3y = 4 ...(ii)
Solution:
Multiply equation (i) by ______ and equation (ii) by ______.
`square` × (3x + 2y = 11) ∴ 9x + 6y = 33 ...(iii)
`square` × (2x + 3y = 4) ∴ 4x + 6y = 8 ...(iv)
Subtract (iv) from (iii),
`square` x = 25
∴ x = `square`
Solve the following pair of equations:
`x/3 + y/4 = 4, (5x)/6 - y/4 = 4`
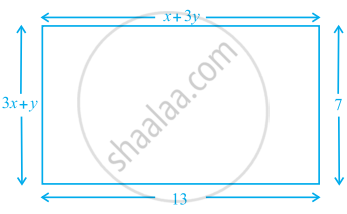
Solve the following pair of equations:
43x + 67y = – 24, 67x + 43y = 24
Anuj had some chocolates, and he divided them into two lots A and B. He sold the first lot at the rate of ₹ 2 for 3 chocolates and the second lot at the rate of ₹ 1 per chocolate, and got a total of ₹ 400. If he had sold the first lot at the rate of ₹ 1 per chocolate, and the second lot at the rate of ₹4 for 5 chocolates, his total collection would have been ₹460. Find the total number of chocolates he had.
