Advertisements
Advertisements
प्रश्न
State and explain the law used to determine the magnetic field at a point due to a current element. Derive the expression for the magnetic field due to a circular current-carrying loop of radius r at its center.
उत्तर
The magnetic field at a certain point due to an element of a current-carrying conductor is given by Biot Savart's law. According to Biot-Savart’s law, the magnitude of the magnetic field dB due to a current-carrying element of length |dl| at a distance r is proportional to the current I, length of the element |dl|, and inversely proportional to the square of the distance r. Its direction is perpendicular to the plane containing dl and r.
Thus, in vector notation,
` "dB" alpha (vec("idl") xx vec("r"))/"r"^3 `
`"dB" = mu_° /(4pi) (vec("idl") xx "r")/"r"^3`
Magnetic field due to a circular current carrying loop:
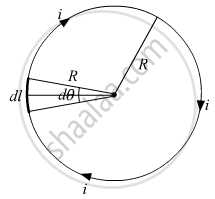
Let the current in the circular loop of radius R is i, now if take a small element of the loop, dl, making an angle dθ at the center.
The magnetic field at the center by using Biot-Savart law is, `vec"dB" = mu_°/(4pi) (vec"idl" xx vec"R")/("R"^3) = mu_°/(4pi) ("idl")/"R"^2 hat"n"` ...`(vec"dl" ⊥ vec"R", hat"n" ⊥ vec "dl"& vec"R")`
∵ dl = rdθ
⇒ `|vec"dB"| = mu_° /(4pi) ("Rd"theta)/R^2 = (mu_°"i")/(4pi"R")"d"theta`
⇒ `|vec"B"| = mu_° /(4pi"R") int_0^(2pi) "d"theta = (mu_°"i")/(2"R")`
⇒ `|vec"B"| = (mu_°"i")/(2"R")`
⇒ `vec"B" = (mu_°"i")/(2"R") hat"n"` ....(Where `hat"n"` is a vector perpendicular to the plane of loop, directed downward)
APPEARS IN
संबंधित प्रश्न
An alpha particle is projected vertically upward with a speed of 3.0 × 104 km s−1 in a region where a magnetic field of magnitude 1.0 T exists in the direction south to north. Find the magnetic force that acts on the α-particle.
The magnetic field at the origin due to a current element \[i d \vec{l}\] placed at a position \[\vec{r}\] is
(a)\[\frac{\mu_0 i}{4\pi}\frac{d \vec{l} \times \vec{r}}{r^3}\]
(b) \[- \frac{\mu_0 i}{4\pi}\frac{\vec{r} \times d \vec{l}}{r^3}\]
(c) \[\frac{\mu_0 i}{4\pi}\frac{\vec{r} \times d \vec{l}}{r^3}\]
(d) \[- \frac{\mu_0 i}{4\pi}\frac{d \vec{l} \times \vec{r}}{r^3}\]
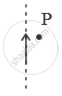
An electric current I flows through an infinitely long conductor as shown in Figure 2 (a) below. Write an expression and direction for the magnetic field at point P.
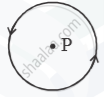
An electric current I flows through a circular loop as shown in Figure 2(b) below. Write an expression and direction for the magnetic field at the centre of the loop at point P.
State and explain the law used to determine magnetic field at a point due to a current element. Derive the expression for the magnetic field due to a circular current carrying loop of radius r at its centre.
State Biot Savart law.
Two concentric circular loops of radius 1 cm and 20 cm are placed coaxially.
(i) Find mutual inductance of the arrangement.
(ii) If the current passed through the outer loop is changed at a rate of 5 A/ms, find the emf induced in the inner loop. Assume the magnetic field on the inner loop to be uniform.
Biot-Savart law indicates that the moving electrons (velocity v) produce a magnetic field B such that ______.
Biot-Savart law indicates that the moving electrons velocity (V) produce a magnetic field B such that ______.
A current carrying loop consists of 3 identical quarter circles of radius R, lying in the positive quadrants of the x-y, y-z and z-x planes with their centres at the origin, joined together. Find the direction and magnitude of B at the origin.
