Advertisements
Advertisements
प्रश्न
Biot-Savart law indicates that the moving electrons velocity (V) produce a magnetic field B such that ______.
विकल्प
- B ∥ v.
- B ⊥ v.
- it obeys inverse cube law.
- it is along the line joining electron and point of observation.
उत्तर
Biot-Savart law indicates that the moving electrons velocity (V) produce a magnetic field B such that B ⊥ v.
Explanation:
According to the Niot-Savart law, the magnitude of `vecB` is: `B oo |q|; B oo v; B oo sin phi; B oo 1/r^2`
`B oo (|q| v sin phi)/r^2`
`B = (mu_0 |q| v sin phi)/r^2`
Where `mu_0/(4pi)` is a proportionality constant, 'r' is the magnitude of position vector from charge to that point at which we have to find the magnetic field and `pgi` is the angle between `vecv` and `vecr`.
or `vecB = (mu_0)/(4pi) (|q| (vecv xx vecr))/(|r^3|) hatn`
Where `hatn` is the direction of `vecB` which is in the direction of cross product of `vecv` and `vecr`. Or we can say that `vecB` ⊥ to both `vecv` and `vecr`.
Where is a proportionality constant, V’ is the magnitude of position vector from charge to that point at which we have to find the magnetic field and < f) is the angle between v and F.
Where h is the direction of B which is in the direction of cross product of v and F . Or we can say that B ⊥ to both v and F.
APPEARS IN
संबंधित प्रश्न
State Biot – Savart law.
Using Biot − Savart’s law, derive the expression for the magnetic field in the vector form at a point on the axis of a circular current loop?
What does a toroid consist of? Find out the expression for the magnetic field inside a toroid for N turns of the coil having the average radius r and carrying a current I. Show that the magnetic field in the open space inside and exterior to the toroid is zero.
State Biot-Savart law.
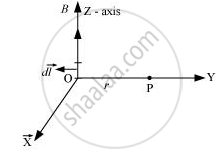
A current I flows in a conductor placed perpendicular to the plane of the paper. Indicate the direction of the magnetic field due to a small element d `vecl` at point P situated at distance `vecr` from the element as shown in the figure.
A long, vertical wire carrying a current of 10 A in the upward direction is placed in a region where a horizontal magnetic field of magnitude 2.0 × 10−3 T exists from south to north. Find the point where the resultant magnetic field is zero.
A regular polygon of n sides is formed by bending a wire of total length 2πr which carries a current i. (a) Find the magnetic filed B at the centre of the polygon. (b) By letting n → ∞, deduce the expression for the magnetic field at the centre of a circular current.
A long wire with a small current element of length 1 cm is placed at the origin and carries a current of 10 A along the X-axis. Find out the magnitude and direction of the magnetic field due to the element on the Y-axis at a distance 0.5 m from it.
Derive the expression for the magnetic field due to a current carrying coil of radius r at a distance x from the centre along the X-axis.
Biot-Sawart law indicates that the moving electrons (velocity `overset(->)("v")`) produce a magnetic field B such that
A current carrying loop consists of 3 identical quarter circles of radius R, lying in the positive quadrants of the x-y, y-z and z-x planes with their centres at the origin, joined together. Find the direction and magnitude of B at the origin.
