Advertisements
Advertisements
प्रश्न
The number of solid spheres, each of diameter 6 cm that can be made by melting a solid metal cylinder of height 45 cm and diameter 4 cm, is:
विकल्प
3
5
4
6
उत्तर
Let r and h be the radius and the height of the cylinder, respectively.
Given:
Diameter of the cylinder = 4 cm
∴ Radius of the cylinder, r = 2 cm
Height of the cylinder, h = 45 cm
Volume of the solid cylinder = `pir^2h=pixx(2)^2xx45 cm^2=180pi cm^3`
Suppose the radius of each sphere be R cm.
Diameter of the sphere = 6 cm
∴ Radius of the sphere, R = 3 cm
Let n be the number of solids formed by melting the solid metallic cylinder.
∴ n × Volume of the solid spheres = Volume of the solid cylinder
`=>nxx4/3 pi R^3=180pi`
`=>nxx4/3 pi(3)^3=180pi`
`=>n=(180xx3)/(4xx27)=5`
Thus, the number of solid spheres that can be formed is 5.
Hence, the correct option is B.
APPEARS IN
संबंधित प्रश्न
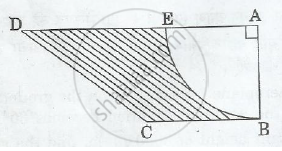
In Figure 2, ABCD is a trapezium of area 24.5 sq. cm. In it, AD|| BC, ∠ DAB = 900, AD = 10 cm and BC = 4 cm. If ABE is a quadrant of a circle, find the area of the shaded region. [Take π=22/7]
2 cubes each of volume 64 cm3 are joined end to end. Find the surface area of the resulting cuboid.
From a solid right circular cylinder of height 2.4 cm and radius 0.7 cm, a right circular cone of same height and same radius is cut out. Find the total surface area of the remaining solid.
A tent of height 77dm is in the form a right circular cylinder of diameter 36m and height 44dm surmounted by a right circular cone. Find the cost of canvas at Rs.3.50 per m2 ?
From a solid cube of side 7 cm , a conical cavity of height 7 cm and radius 3 cm is hollowed out . Find the volume of the remaining solid.
Volume and surface area of a solid hemisphere are numerically equal. What is the diameter of hemisphere?
A solid is hemispherical at the bottom and conical above. If the surface areas of the two parts are equal, then the ratio of its radius and the height of its conical part is
A solid sphere of radius r is melted and cast into the shape of a solid cone of height r, the radius of the base of the cone is
The shape of the gilli used in a gilli-danda game is a combination of
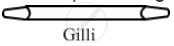
The radius of spherical balloon increases from 8 cm to 12 cm. The ratio of the surface areas of balloon in two cases is ______.
