Advertisements
Advertisements
प्रश्न
The perimeter of a triangle ABC is 37 cm and the ratio between the lengths of its altitudes be 6: 5: 4. Find the lengths of its sides.
Let the sides be x cm, y cm, and (37 - x - y) cm. Also, let the lengths of altitudes be 6a cm, 5a cm, and 4a cm.
उत्तर
Consider that the sides be x cm, y cm, and (37 - x - y) cm. also, consider that the lengths of altitudes be 6a cm, 5a cm, and 4a cm.
∴ Area of a triangle = `1/2` x base x altitude
∴ `1/2 xx x xx 6a = 1/2 xx y xx 5a = 1/2 xx ( 37 - x - y) xx 4a`
6x = 5y = 148 - 4x - 4y
6x = 5y and 6x = 148 - 4x - 4y
6x - 5y = 0 and 10x + 4y = 148
Solving both the equations, we have
X = 10 cm, y = 12 cm and ( 37 - x - y ) cm = 15cm.
APPEARS IN
संबंधित प्रश्न
In the below Fig, ABC and ABD are two triangles on the base AB. If line segment CD is
bisected by AB at O, show that ar (Δ ABC) = ar (Δ ABD)

ABCD is a parallelogram whose diagonals AC and BD intersect at O. A line through O
intersects AB at P and DC at Q. Prove that ar (Δ POA) = ar (Δ QOC).
Two parallelograms are on the same base and between the same parallels. The ratio of their areas is
In a ΔABC if D and E are mid-points of BC and AD respectively such that ar (ΔAEC) = 4cm2, then ar (ΔBEC) =
The medians of a triangle ABC intersect each other at point G. If one of its medians is AD,
prove that:
(i) Area ( ΔABD ) = 3 x Area ( ΔBGD )
(ii) Area ( ΔACD ) = 3 x Area ( ΔCGD )
(iii) Area ( ΔBGC ) = `1/3` x Area ( ΔABC ).
So the area of piece A = ________ square cm
Nasreena is a farmer who wants to divide her land equally among her three children — Chumki, Jhumri, and Imran. She wants to divide the land so that each piece of land has one tree. Her land looks like this.
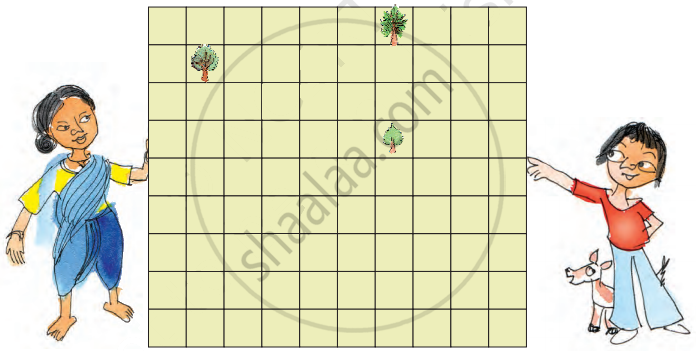
- Can you divide the land equally? Show how you will divide it. Remember each person has to get a tree. Colour each person’s piece of land differently.
Find all the possible dimensions (in natural numbers) of a rectangle with a perimeter 36 cm and find their areas.
How will you decide? Discuss.
Find the area of the following figure by counting squares:

