Advertisements
Advertisements
प्रश्न
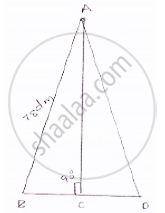
The perimeter of a triangular field is 240 dm. If two of its sides are 78 dm and 50 dm, find the length of the perpendicular on the side of length 50 dm from the opposite vertex.
उत्तर
ABC be the triangle, Here a = 78 dm = AB,
BC = b = 50 dm
Now, perimeter = 240 dm
⇒ AB + BC + CA = 240 dm
⇒ AC = 240 – BC – AB
⇒ AC = 112 dm
Now, 2s = AB + BC + CA
⇒ 2s = 240
⇒ s = 120 dm
∴ Area of ΔABC =`sqrt(s(s-a)(s-b)(s-b))` by heron's formula
=`sqrt(120(120-78)(120-50)(120-112))`
`=sqrt(120xx42xx70xx8)`
1680 `dm^2`
𝐿𝑒𝑡 𝐴𝐷 𝑏𝑒 𝑝𝑒𝑟𝑝𝑒𝑛𝑑𝑖𝑐𝑢𝑎𝑙𝑎𝑟 𝑜𝑛 𝐵𝐶
Area of ΔABC = `1/2xx ABxxBC `(area of triangle=`(1/2xxbxxh)`
`=1/2xx ADxxBC=1680 `
`⇒AD=(2xx1680)/50=67.2dm`
APPEARS IN
संबंधित प्रश्न
A traffic signal board, indicating ‘SCHOOL AHEAD’, is an equilateral triangle with side ‘a’. Find the area of the signal board, using Heron’s formula. If its perimeter is 180 cm, what will be the area of the signal board?
An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
A triangle has sides 35 cm, 54 cm and 61 cm long. Find its area. Also, find the smallest of its altitudes ?
A triangle and a parallelogram have the same base and the same area. If the sides of the triangle are 13 cm, 14 cm and 15 cm and the parallelogram stands on the base 14 cm, find the height of the parallelogram.
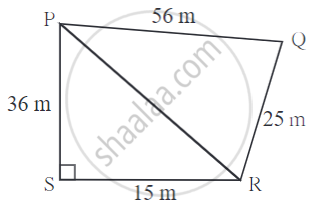
Look at the measures shown in the adjacent figure and find the area of ☐ PQRS.
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
A triangular shaped glass with vertices at A(– 5, – 4), B(1, 6) and C(7, – 4) has to be painted. If one bucket of paint covers 6 square feet, how many buckets of paint will be required to paint the whole glass, if only one coat of paint is applied
Using Heron’s formula, find the area of a triangle whose sides are 10 cm, 24 cm, 26 cm
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13 m, 14 m and 15 m. The advertisements yield an earning of Rs 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
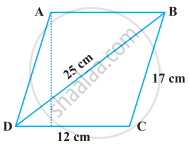
Find the area of a parallelogram given in figure. Also find the length of the altitude from vertex A on the side DC.