Advertisements
Advertisements
प्रश्न
Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of radius 5m drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma. If the distance between Reshma and Salma and between Salma and Mandip is 6m each, what is the distance between Reshma and Mandip?
उत्तर
Draw perpendiculars OA and OB on RS and SM, respectively.
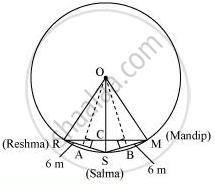
AR = AS = `6/2` = 3m
OR = OS = OM = 5m (Radii of the circle)
In ΔOAR,
OA2 + AR2 = OR2
OA2 + (3m)2 = (5m)2
OA2 = (25 − 9) m2 = 16m2
OA = 4m
ORSM will be a kite (OR = OM and RS = SM). We know that the diagonals of a kite are perpendicular and the diagonal common to both isosceles triangles is bisected by another diagonal.
∴ ∠RCS will be of 90° and RC = CM
Area of ΔORS = `1/2 xx OA xx RS`
`1/2 xx RC xx OS` = `1/2 xx 4 xx 6`
Rc × 5 = 24
RC = 4.8
RM = 2RC
RM = 2(4.8)
RM = 9.6
Therefore, the distance between Reshma and Mandip is 9.6 m.
APPEARS IN
संबंधित प्रश्न
If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C, and D, prove that AB = CD (see given figure).

true or false
If a circle is divided into three equal arcs each is a major arc.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle ofradius 10 cm.
Two equal circles of radius r intersect such that each passes through the centre of the other. The length of the common chord of the circle is
Two chords AB and CD of a circle are each at distances 4 cm from the centre. Then AB = CD.
Two chords AB and AC of a circle with centre O are on the opposite sides of OA. Then ∠OAB = ∠OAC .
Two congruent circles with centres O and O′ intersect at two points A and B. Then ∠AOB = ∠AO′B.
AB and AC are two chords of a circle of radius r such that AB = 2AC. If p and q are the distances of AB and AC from the centre, prove that 4q2 = p2 + 3r2.
