Advertisements
Advertisements
प्रश्न
Two chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the bisector of angle BAC.
उत्तर
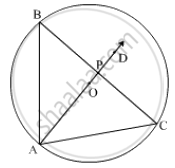
Given: AB and AC are two equal chords of C (O, r).
To prove: Centre, O lies on the bisector of ∠BAC.
Construction: Join BC. Let the bisector of ∠BAC intersects BC in P.
Proof:
In ΔAPB and ΔAPC,
AB = AC ...(Given)
∠BAP = ∠CAP ...(Given)
AP = AP ...(Common)
∴ ΔAPB ≅ ΔAPC ...(SAS congruence criterion)
`=>` BP = CP and ∠APB = ∠APC ...(CPCT)
∠APB + ∠APC = 180° ...(Linear pair)
`=>` 2∠APB = 180° ...(∠APB = ∠APC)
`=>` ∠APB = 90°
Now, BP = CP and ∠APB = 90°
∴ AP is the perpendicular bisector of chord BC.
`=>` AP passes through the centre, O of the circle.
APPEARS IN
संबंधित प्रश्न
The radius of a circle is 17.0 cm and the length of perpendicular drawn from its centre to a chord is 8.0 cm. Calculate the length of the chord.
A chord CD of a circle whose centre is O, is bisected at P by a diameter AB.
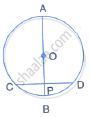
Given OA = OB = 15 cm and OP = 9 cm. calculate the length of:
(i) CD (ii) AD (iii) CB
PQ and QR are two equal chords of a circle. A diameter of the circle is drawn through Q . Prove that the diameter bisects ∠ PQR.
From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:
OP is the ⊥ bisector of chord AB.
A chord CD of a circle whose center is O is bisected at P by a diameter AB. Given OA = OB = 15 cm and OP = 9 cm.
Calculate the lengths of: (i) CD ; (ii) AD ; (iii) CB.
A chord of length 6 cm is drawn in a circle of radius 5 cm.
Calculate its distance from the center of the circle.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
Two chords AB and CD of a circle are parallel and a line L is the perpendicular bisector of AB. Show that L bisects CD.
AB is a diameter of a circle with centre C = (- 2, 5). If A = (3, – 7). Find
(i) the length of radius AC
(ii) the coordinates of B.
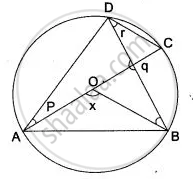
In the figure, AC is the diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q, r in term of x.