Advertisements
Advertisements
प्रश्न
Which of the following combinations should be selected for better tuning of an LCR circuit used for communication?
विकल्प
R = 20 Ω, L = 1.5 H, C = 35µF.
R = 25 Ω, L = 2.5 H, C = 45µF.
R = 15 Ω, L = 3.5 H, C = 30µF.
R = 25 Ω, L = 1.5 H, C = 45µF.
उत्तर
R = 15 Ω, L = 3.5 H, C = 30µF.
Explanation:
Quality factor (Q-factor) of the series resonant circuit:
(i) The characteristic of a series resonant circuit is determined by the quality factor (Q-factor) of the circuit.
(ii) It defines sharpness if i - v curve at resonance when Q-factor is large, the sharpness of resonance curve is more and vice-versa.
(iii) Q-factor is also defined as follows:
Q-factor = `2pi xx "Max energy stored"/"Energy dissipation"`
= `(2pi)/T xx "Max energy stored"/"Mean power dissipated"`
= `"Resonant frequency"/"Bandwidth" = ω_0/(Δω)`
(iv) Q-factor = `V_L/V_R` or `V_C/V_R = (ω_0L)/R` or `1/(ω_0CR)`
⇒ Q-factor = `1/R sqrt(L/C)`
For better tuning of an L-C-R circuit used for communication, quality factor of the circuit must be as high as possible.
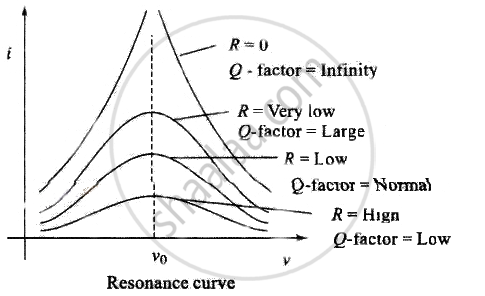
We know quality factor should be high for better tuning.
Quality factor (Q) of an L-C-R circuit is `Q = 1/R sqrt(L/C)`
Where R is the resistance, L is the inductance and C is the capacitance of the circuit.
For high Q factor R should be low, L should be high and C should be low.
Important point: Be careful while writing formula for quality factor, this formula we used in this case is only for series L-C-R circuit.
APPEARS IN
संबंधित प्रश्न
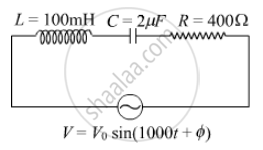
(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
(ii) Without making any other change, find the value of the additional capacitor C1, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
An L-R circuit has L = 1.0 H and R = 20 Ω. It is connected across an emf of 2.0 V at t = 0. Find di/dt at (a) t = 100 ms, (b) t = 200 ms and (c) t = 1.0 s.
An inductor-coil of inductance 17 mH is constructed from a copper wire of length 100 m and cross-sectional area 1 mm2. Calculate the time constant of the circuit if this inductor is joined across an ideal battery. The resistivity of copper = 1.7 × 10−8 Ω-m.
A constant current exists in an inductor-coil connected to a battery. The coil is short-circuited and the battery is removed. Show that the charge flown through the coil after the short-circuiting is the same as that which flows in one time constant before the short-circuiting.
Answer the following question.
In a series LCR circuit connected across an ac source of variable frequency, obtain the expression for its impedance and draw a plot showing its variation with frequency of the ac source.
In an L.C.R. series a.c. circuit, the current ______.
Which of the following components of an LCR circuit, with a.c. supply, dissipates energy?
Define Impedance.
A series RL circuit with R = 10 Ω and L = `(100/pi)` mH is connected to an ac source of voltage V = 141 sin (100 πt), where V is in volts and t is in seconds. Calculate
- the impedance of the circuit
- phase angle, and
- the voltage drop across the inductor.
Three students, X, Y and Z performed an experiment for studying the variation of a.c. with frequency in a series LCR circuit and obtained the graphs as shown below. They all used
- an AC source of the same emf and
- inductance of the same value.
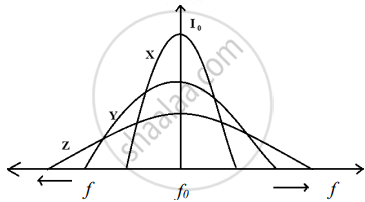
- Who used minimum resistance?
- In which case will the quality Q factor be maximum?
- What did the students conclude about the nature of impedance at resonant frequency (f0)?
- An ideal capacitor is connected across 220V, 50Hz, and 220V, 100Hz supplies. Find the ratio of current flowing through it in the two cases.
