Advertisements
Advertisements
In ∆ABC, AP ⊥ BC, BQ ⊥ AC B– P–C, A–Q – C then prove that, ∆CPA ~ ∆CQB. If AP = 7, BQ = 8, BC = 12 then Find AC.

Concept: undefined > undefined
Select the appropriate alternative.
In ∆ABC and ∆PQR, in a one to one correspondence \[\frac{AB}{QR} = \frac{BC}{PR} = \frac{CA}{PQ}\]

Concept: undefined > undefined
Advertisements
If in ∆DEF and ∆PQR, ∠D ≅ ∠Q, ∠R ≅ ∠E then which of the following statements is false?

Concept: undefined > undefined
In ∆ABC and ∆DEF ∠B = ∠E, ∠F = ∠C and AB = 3DE then which of the statements regarding the two triangles is true ?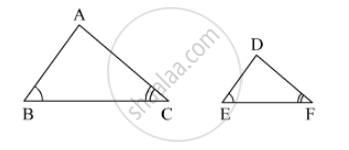
Concept: undefined > undefined
∆ABC and ∆DEF are equilateral triangles, A(∆ABC): A(∆DEF) = 1: 2. If AB = 4 then what is length of DE?

Concept: undefined > undefined
In the given figure, seg XY || seg BC, then which of the following statements is true?

Concept: undefined > undefined
In the given figure, A – D – C and B – E – C seg DE || side AB If AD = 5, DC = 3, BC = 6.4 then Find BE.

Concept: undefined > undefined
Find the ratio in which the line segment joining the points A(3, 8) and B(–9, 3) is divided by the Y– axis.
Concept: undefined > undefined
Find the coordinates of the circumcentre of a triangle whose vertices are (–3, 1), (0, –2) and (1, 3).
Concept: undefined > undefined
Find the coordinates of circumcentre and radius of circumcircle of ∆ABC if A(7, 1), B(3, 5) and C(2, 0) are given.
Concept: undefined > undefined
Find the coordinates of the centre of the circle passing through the points P(6, –6), Q(3, –7) and R (3, 3).
Concept: undefined > undefined
Find the possible pairs of coordinates of the fourth vertex D of the parallelogram, if three of its vertices are A(5, 6), B(1, –2) and C(3, –2).
Concept: undefined > undefined
Ponit M is the mid point of seg AB and AB = 14 then AM = ?
Concept: undefined > undefined

Observe the adjoining figure and write down one pair of interior angles.
Concept: undefined > undefined
If Δ ABC ∼ Δ XYZ then complete the following brackets.
`(AB)/(XY) = /(YZ) = (AC)/`
Concept: undefined > undefined
Draw ∠ ARP= 115° and bisect it.
Concept: undefined > undefined
Write down the equation of X- axis.
Concept: undefined > undefined
Show that A(-4, -7), B(-1, 2), C(8, 5) and D(5, -4) are the vertices of a
rhombus ABCD.
Concept: undefined > undefined
In the figure, parts of the two triangles bearing identical marks are
congruent. State the test by which the triangles are congruent.

Concept: undefined > undefined
Show that `square` ABCD formed by the vertices A(-4,-7), B(-1,2), C(8,5) and D(5,-4) is a rhombus.
Concept: undefined > undefined
