मराठी
CUET (UG)
MCQ ऑनलाइन सराव परीक्षा१४८
संकल्पना स्पष्टीकरण२८६
अभ्यासक्रम

Equation of a Plane - Plane Passing Through the Intersection of Two Given Planes

Advertisements

Topics

Notes

Let `π_1` and `π_2` be two planes with equations `vec r  . hat n _1 = d_1` and `vec r . hat n _2 = d_2` respectively.  The position vector of any point on the line of intersection must satisfy both the equations fig.

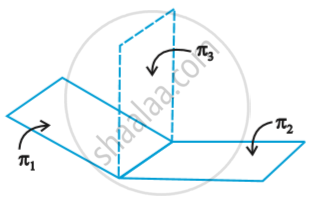
If `vec t ` is the position vector of a point on the line , then
`vec t . hat n_1 = d_1` and `vec t . hat n _2 = d_2` 
Therefore , for all real values of  λ, we have
`vec t . (hat n _1 + lambda hat n_2) = d_1 + lambda d_2`
Since `vec t` is arbitrary, it satisfies for any point on the line.
Hence , the equation `vec r . (vec n_1 + lambda vec n_2) = d_1 + lambda d_2`   represents a plane `π_3` which is such  that if any vector ` vec r` satisfies both the equations `π_1` and `π_2`, it also satisfies the equation `π_3` i.e., any plane passing through the intersection of the planes 
`vec r . vec n_1 = d_1` and `vec r . vec n_2 = d_2`
has the equation  `vec r . (vec n_1 + lambda vec n_2) = d_1 + lambda d_2`    ...(1)

Cartesian form:
In Cartesian system, let  `vec n_1 = A_1 hat i + B_2 hat j + C_1 hat k`
`vec n_2 = A_2 hat i + B_2 hat j + C _2 hat k` 
and `vec r = x hat i + y hat j + z hat k`
Then (1) becomes 
`x (A_1 + lambda A_2) + y(B_1 + lambda B_2) + z(C_1 +lambda C_2) = d_1 + lambda d_2`
or `(A_1x +B_1y + C_1z -d_1) + lambda (A_2x + B_2y + C_2z -d_2) = 0`     ..(2)
which is the required Cartesian form of the equation of the plane passing through the intersection of the given planes for each value of λ.

If you would like to contribute notes or other learning material, please submit them using the button below.

Related QuestionsVIEW ALL [40]

Find the distance of the point P(−1, −5, −10) from the point of intersection of the line joining the points A(2, −1, 2) and B(5, 3, 4) with the plane  \[x - y + z = 5\] . 

 

Find the equation of the plane through the intersection of the planes 3x – y + 2z – 4 = 0 and x + y + z – 2 = 0 and the point (2, 2, 1).

A vector parallel to the line of intersection of the planes\[\vec{r} \cdot \left( 3 \hat{i} - \hat{j} + \hat{k}  \right) = 1 \text{ and }  \vec{r} \cdot \left( \hat{i} + 4 \hat{j}  - 2 \hat{k}  \right) = 2\] is 

 

Find the vector equation of the plane passing through three points with position vectors  \[\hat{i}  + \hat{j}  - 2 \hat{k}  , 2 \hat{i}  - \hat{j}  + \hat{k}  \text{ and }  \hat{i}  + 2 \hat{j}  + \hat{k}  .\]  Also, find the coordinates of the point of intersection of this plane and the line  \[\vec{r} = 3 \hat{i}  - \hat{j}  - \hat{k}  + \lambda\left( 2 \hat{i}  - 2 \hat{j} + \hat{k} \right) .\]

 

Find the equation of the plane through the intersection of the planes `vec"r" * (hat"i" + 3hat"j") - 6` = 0 and `vec"r" * (3hat"i" - hat"j" - 4hat"k")` = 0, whose perpendicular distance from origin is unity.

The equation of the curve passing through the point `(0, pi/4)` whose differential equation is sin x cos y dx + cos x sin y dy = 0, is

Find the equation of the plane which is perpendicular to the plane 5x + 3y + 6z + 8 = 0 and which contains the line of intersection of the planes x + 2y + 3z – 4 = 0 and 2x + y – z + 5 = 0.

Show that the lines `(x - 1)/2 = (y - 2)/3 = (z - 3)/4` and `(x - 4)/5 = (y - 1)/2` = z intersect. Also, find their point of intersection.

Related concepts

Advertisements
Share
Notifications

Englishहिंदीमराठी
Login
Create free account


      Forgot password?
Course
Use app×