Topics
Roman Numerals
Number Work
Addition and Subtraction
Multiplication and Division
Fractions
Angles
Circles
Multiples and Factors
Decimal Fractions
- Decimal Fractions
- The Decimal Number System
- Concept of Tenths, Hundredths and Thousandths in Decimal
- Concept of Place Value
- Use of Decimal Fraction
- Writing Half, Quarter, Three-quarters and One and a Quarter in Decimal Form
- Addition of Decimal Fraction
- Subtraction of Decimal Fraction
- Decimals Used for Measurement
Measuring Time
Problems on Measurement
Perimeter and Area
Three Dimensional Objects and Nets
Pictographs
Patterns
Preparation for Algebra
Pairing off objects from two groups in different ways
"Pairing off objects from two groups in different ways" means finding all possible combinations of pairing one object from one group with another object from a second group.
The number of different pairs formed by pairing off objects from two groups is equal to the product of the number of objects in the two groups.
Examples:
(1) Ajay wants to travel light. So he took with him three shirts—one red, one green, and one blue—and two pairs of trousers—one white and one black. How many different ways does he have of pairing off a shirt with trousers?
Writing ‘S’ for shirt and ‘T’ for trousers, the possible different pairs are:
- (Red S, Black T)
- (Green S, Black T)
- (Blue S, Black T)
- (Red S, White T)
- (Green S, White T)
- (Blue S, White T)
(2) Suresh has three balls of different colours marked A, B, and C, and three bats marked P, Q, and R. He wishes to take only one bat and one ball to the playground. In how many ways can he pair off a ball and a bat to take with him?
How many different pairs have been shown here?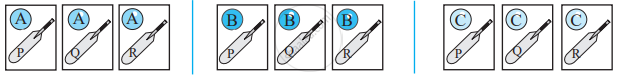
Suresh has three bats (P, Q, and R) and three balls (A, B, and C). He wants to go to the playground with just one bat and one ball.
We apply the multiplication principle to determine how many ways he can combine a ball with a bat:
So, the total number of different pairs he can make is 9.
The different pairs are:
