Advertisements
Advertisements
प्रश्न
5 g of a gas is contained in a rigid container and is heated from 15°C to 25°C. Specific heat capacity of the gas at constant volume is 0.172 cal g−1 °C−1 and the mechanical equivalent of heat is 4.2 J cal−1. Calculate the change in the internal energy of the gas
उत्तर
Given:
Mass of the gas, m = 5 g
Change in temperature of the system, ∆T = 25 − 15°C = 10°C
Specific heat at constant volume, Cv = 0.172 cal/g -°C
Mechanical equivalent, J = 4.2 J/cal
From the first law of thermodynamics,
dQ = dU +dW
Now ,
ΔV = 0 (Rigid wall of the container keeps the volume constant)
So, dW =PΔV =0
Therefore,
dQ =dU (From the first law)
Q = mcvdT = 5×0.172×10
= 8.6 cal = 8.6 × 4.2 J
= 36.12 J
So, change in internal energy of the system is 36.12 J.
APPEARS IN
संबंधित प्रश्न
A metre long narrow bore held horizontally (and closed at one end) contains a 76 cm long mercury thread, which traps a 15 cm column of air. What happens if the tube is held vertically with the open end at the bottom?
Given below are densities of some solids and liquids. Give rough estimates of the size of their atoms:
| Substance | Atomic Mass (u) | Density (103 Kg m-3) |
| Carbon (diamond) | 12.01 | 2.22 |
| Gold | 197.00 | 19.32 |
| Nitrogen (liquid) | 14.01 | 1.00 |
| Lithium | 6.94 | 0.53 |
| Fluorine (liquid) | 19.00 | 1.14 |
[Hint: Assume the atoms to be ‘tightly packed’ in a solid or liquid phase, and use the known value of Avogadro’s number. You should, however, not take the actual numbers you obtain for various atomic sizes too literally. Because of the crudeness of the tight packing approximation, the results only indicate that atomic sizes are in the range of a few Å].
The specific heat capacity of water is
Can we define specific heat capacity for an adiabatic process?
Does a solid also have two kinds of molar heat capacities Cp and Cv? If yes, is Cp > Cv? Or is Cp − Cv = R?
In a real gas, the internal energy depends on temperature and also on volume. The energy increases when the gas expands isothermally. Examining the derivation of Cp − Cv = R, find whether Cp − Cv will be more than R, less than R or equal to R for a real gas.
Can a process on an ideal gas be both adiabatic and isothermal?
Show that the slope of the p−V diagram is greater for an adiabatic process compared to an isothermal process.
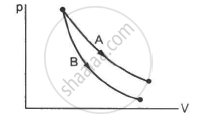
Two samples A and B are initially kept in the same state. Sample A is expanded through an adiabatic process and the sample B through an isothermal process. The final volumes of the samples are the same. The final pressures in A and B are pA and pBrespectively.
Let ∆Wa and ∆Wb be the work done by the systems A and B, respectively, in the previous question.
Consider the processes A and B shown in the figure. It is possible that
A mixture contains 1 mole of helium (Cp = 2.5 R, Cv = 1.5 R) and 1 mole of hydrogen (Cp= 3.5 R, Cv = 2.5 R). Calculate the values of Cp, Cv and γ for the mixture.
Air (γ = 1.4) is pumped at 2 atm pressure in a motor tyre at 20°C. If the tyre suddenly bursts, what would be the temperature of the air coming out of the tyre? Neglect any mixing with the atmospheric air.
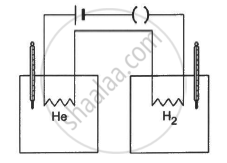
The figure shows two vessels with adiabatic walls, one containing 0.1 g of helium (γ = 1.67, M = 4 g mol−1) and the other containing some amount of hydrogen (γ = 1.4, M = 2 g mol−1). Initially, the temperatures of the two gases are equal. The gases are electrically heated for some time during which equal amounts of heat are given to the two gases. It is found that the temperatures rise through the same amount in the two vessels. Calculate the mass of hydrogen.
The speed of sound in hydrogen at 0°C is 1280 m s−1. The density of hydrogen at STP is 0.089 kg m−3. Calculate the molar heat capacities Cp and Cv of hydrogen.
4.0 g of helium occupies 22400 cm3 at STP. The specific heat capacity of helium at constant pressure is 5.0 cal K−1 mol−1. Calculate the speed of sound in helium at STP.
Standing waves of frequency 5.0 kHz are produced in a tube filled with oxygen at 300 K. The separation between the consecutive nodes is 3.3 cm. Calculate the specific heat capacities Cp and Cv of the gas.
A diatomic molecule can be modelled as two rigid balls connected with spring such that the balls can vibrate with respect to centre of mass of the system (spring + balls). Consider a diatomic gas made of such diatomic molecule. If the gas performs 20 Joule of work under isobaric condition, then heat given to the gas is ______ J.
