Advertisements
Advertisements
प्रश्न
A 600 pF capacitor is charged by a 200 V supply. It is then disconnected from the supply and is connected to another uncharged 600 pF capacitor. How much electrostatic energy is lost in the process?
उत्तर
Capacitance of the capacitor, C = 600 pF
Potential difference, V = 200 V
Electrostatic energy stored in the capacitor is given by,
`"E" = 1/2 "CV"^2`
= `1/2 xx (600 xx 10^-12) xx (200)^2`
= 1.2 × 10−5 J
If supply is disconnected from the capacitor and another capacitor of capacitance C = 600 pF is connected to it, then equivalent capacitance (C') of the combination is given by,
`1/"C'" = 1/"C" + 1/"C"`
= `1/600 + 1/600`
= `2/600`
= `1/300`
C' = 300 pF
New electrostatic energy can be calculated as
`"E'" = 1/2 xx "C'" xx "V"^2`
= `1/2 xx 300 xx (200)^2`
= 0.6 × 10−5 J
Loss in electrostatic energy = E − E'
= 1.2 × 10−5 − 0.6 × 10−5
= 0.6 × 10−5
= 6 × 10−6 J
Therefore, the electrostatic energy lost in the process is 6 × 10−6 J.
APPEARS IN
संबंधित प्रश्न
Obtain the expression for the energy stored per unit volume in a charged parallel plate capacitor.
A 12 pF capacitor is connected to a 50 V battery. How much electrostatic energy is stored in the capacitor?
Find the ratio of energy stored in the two configurations if they are both connected to the same source.
The energy density in the electric field created by a point charge falls off with the distance from the point charge as
A capacitor of capacitance 500 μF is connected to a battery through a 10 kΩ resistor. The charge stored in the capacitor in the first 5 s is larger than the charge stored in the next.
(a) 5 s
(b) 50 s
(c) 500 s
(d) 500 s
Find the charge on the capacitor shown in the figure.

A capacitance C, a resistance R and an emf ε are connected in series at t = 0. What is the maximum value of (a) the potential difference across the resistor (b) the current in the circuit (c) the potential difference across the capacitor (d) the energy stored in the capacitor (e) the power delivered by the battery and (f) the power converted into heat?
A capacitance C charged to a potential difference V is discharged by connecting its plates through a resistance R. Find the heat dissipated in one time constant after the connections are made. Do this by calculating ∫ i2R dt and also by finding the decrease in the energy stored in the capacitor.
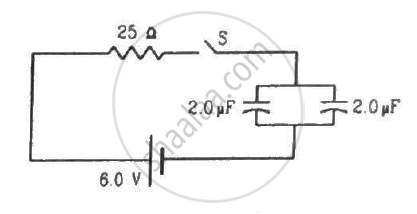
Find the charge on each of the capacitors 0.20 ms after the switch S is closed in the figure.
A capacitor with stored energy 4⋅0 J is connected with an identical capacitor with no electric field in between. Find the total energy stored in the two capacitors.
A capacitor of capacitance 100 μF is connected across a battery of emf 6 V through a resistance of 20 kΩ for 4 s. The battery is then replaced by a thick wire. What will be the charge on the capacitor 4 s after the battery is disconnected?
A capacitor of capacitance C is given a charge Q. At t = 0, it is connected to an uncharged capacitor of equal capacitance through a resistance R. Find the charge on the second capacitor as a function of time.
A metal sphere of radius R is charged to a potential V.
- Find the electrostatic energy stored in the electric field within a concentric sphere of radius 2 R.
- Show that the electrostatic field energy stored outside the sphere of radius 2 R equals that stored within it.
Choose the correct option:
Energy stored in a capacitor and dissipated during charging a capacitor bear a ratio.
A capacitor is a device that stores ____________.
An air-filled parallel plate capacitor has a uniform electric field `overset(->)("E")` in the space between the plates. If the distance between the plates is 'd' and the area of each plate is 'A', the energy stored in the capacitor is ______
(∈0 = permittivity of free space)
What fraction of the energy drawn from the charging battery is stored in a capacitor?
Do free electrons travel to region of higher potential or lower potential?
A parallel plate capacitor (A) of capacitance C is charged by a battery to voltage V. The battery is disconnected and an uncharged capacitor (B) of capacitance 2C is connected across A. Find the ratio of total electrostatic energy stored in A and B finally and that stored in A initially.
Derive an expression for energy stored in a capacitor.
