Advertisements
Advertisements
प्रश्न
A current-carrying circular coil of 100 turns and radius 5.0 cm produces a magnetic field of 6.0 × 10−5 T at its centre. Find the value of the current.
उत्तर
Given:
No. of turns, n = 100
Radius of the loop, r = 5 cm = 0.05 m
Magnetic field intensity, B = 6.0 × 10−5 T
Now, let the magnitude of current be i.
\[\text{ Using } B = \frac{\mu_0 \text{ ni} }{2r}, \text{ we get } \]
\[6 . 0 \times {10}^{- 7} = \frac{4\pi \times {10}^{- 7} \times {10}^2 \times i}{2 \times 0 . 05}\]
\[ \Rightarrow i = \frac{60 \times {10}^{- 7}}{4\pi \times {10}^{- 7} \times {10}^2}\]
\[ = 4 . 777 \times {10}^{- 2} \approx 48\] mA
APPEARS IN
संबंधित प्रश्न
Two identical circular loops, P and Q, each of radius r and carrying equal currents are
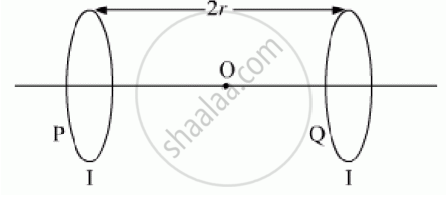
kept in the parallel planes having a common axis passing through O. The direction of current in P is clockwise and in Q is anti-clockwise as seen from O which is equidistant from the loops P and Q. Find the magnitude of the net magnetic field at O.
The torque on a current loop is zero if the angle between the positive normal and the magnetic field is either θ = 0 or θ = 180°. In which of the two orientations, the equilibrium is stable?
A current loop of arbitrary shape lies in a uniform magnetic field B. Show that the net magnetic force acting on the loop is zero.
A circular loop carrying a current i is made of a wire of length L. A uniform magnetic field B exists parallel to the plane of the loop. (a) Find the torque on the loop. (b) If the same length of the wire is used to form a square loop, what would be the torque? Which is larger?
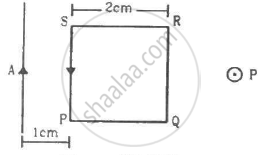
A square loop PQRS carrying a current of 6.0 A is placed near a long wire carrying 10 A as shown in figure. (a) Show that the magnetic force acting on the part PQ is equal and opposite to the part RS. (b) Find the magnetic force on the square loop.
A moving coil galvanometer has been fitted with a rectangular coil having 50 turns and dimensions 5 cm × 3 cm. The radial magnetic field in which the coil is suspended is of 0.05 Wb/m2. The torsional constant of the spring is 1.5 × 10−9 Nm/degree. Obtain the current required to be passed through the galvanometer so as to produce a deflection of 30°.
A Rectangular coil of 10 turns, each of area 0.05 m2, is suspended freely in a uniform magnetic field of induction 0.01 T. A current of 30 µA is passed through it.
(i) What is the magnetic moment of the coil?
(ii) What is the maximum torque experienced by the coil?
Derive an expression for the net torque on a rectangular current carrying loop placed in a uniform magnetic field with its rotational axis perpendicular to the field.
A rectangular coil of length 0.12 m and width 0.1 m having 100 turns of wire is suspended vertically in a uniform magnetic field of strength 0.4 Wb/m2. The coil carries a current of 2.5 A. If the plane of the coil is inclined at an angle of 30° with the direction of the field, the torque required to keep the coil in stable equilibrium will be ____________.
Torque acting on a rectangular coil carrying current 'l' situated parallel to magnetic field of induction 'B', having number of turns 'n' and area 'A' is ______.
A triangular loop of side `l` carries a current I. It is placed in a magnetic field B such that the plane of the loop is in the direction of B. The torque on the loop is ____________.
A small cylindrical soft iron piece is kept in a galvanometer so that
If the net magnetic force acting on a loop is zero then ____________.
Which one of the following statements is 'NOT' TRUE? Sensitivity of a moving coil galvanometer can be increased by ____________.
In suspended type of moving coil galvanometer ____________.
An ammeter is obtained by shunting 'n' `Omega` galvanometer with 'n' `Omega` resistance. The additional shunt required to be connected across it to double the range is ____________.
The initial pressure and volume of a gas enclosed in a cylinder are 2 × 105 N/m2 and 6 × 10-3 m3 respectively. If the work done in compressing the gas at constant pressure is 150 J. find the final volume of the gas.
Equal current i flows in two segments of a circular loop in the direction shown in figure. Radius of the loop is r. The magnitude of magnetic field induction at the centre of the loop is ______.

Write the formula for torque acting on rotating current carrying coil in terms of magnetic dipole moment, in vector form.
An electron moving along positive X axis with a velocity of 8 ×107ms-1 enters a region having uniform magnetic field B = 1.3 × 10-3 T along positive Y axis.
- Explain why the electron describes a circular path.
- Calculate the radius of the circular path described by the electron.
