Advertisements
Advertisements
प्रश्न
A cycle followed by an engine (made of one mole of perfect gas in a cylinder with a piston) is shown in figure.
A to B : volume constant
B to C : adiabatic
C to D : volume constant
D to A : adiabatic
VC = VD = 2VA = 2VB
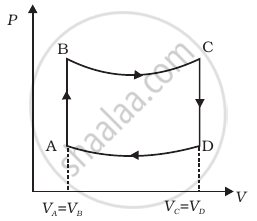
- In which part of the cycle heat is supplied to the engine from outside?
- In which part of the cycle heat is being given to the surrounding by the engine?
- What is the work done by the engine in one cycle? Write your answer in term of PA, PB, VA.
- What is the efficiency of the engine?
(γ = `5/3` for the gas), (Cv = `3/2` R for one mole)
उत्तर
a. For process AB (which is the isochoric process), volume is constant.
So, dV = 0
⇒ dW = 0
dQ = dU + dW = dU
⇒ dQ = dU = Change in internal energy
Hence, in this process heat supplied is utilised to increase, the internal energy of the system.
b. For the process CD (which is also an isochoric process), volume is constant but the pressure decreases. Hence, temperature also decreases (because PαT) so heat is given to the surroundings.
c. To calculate work done by the engine in one cycle, we calculate work done in each part separately.
(i) `W_(AB) = int_A^B PdV` = 0
(ii) `W_(CD) = int_C^D PdV` = 0 ....(As V is constant, dV = 0)
(iii) `W_(BC) = int_B^C PdV`
= `K int_B^C (dV)/(V^γ)`
= `K int_(V_B)^(V_C) V^-γ dV`
= `K/(1 - γ) [V^(1 - γ)]_(V_B)^(V_C)`
= `(K[V_C^(1 - γ) - V_B])/(1 - γ)` .....(PVγ = K for an adiabatic change)
= `([(P_CV_C^γ)(V_C^(1 - γ)) - (P_BV_B^γ)(V_B^(1 - γ))])/((1 - γ))`
Similarly, `W_(DA) = int_(V_D)^(V_A) PdV`
= `1/((1 - γ)) (P_AV_A - P_DV_D)` ......[∵ BC is an adiabatic process]
Since B and C lies on adiabatic curve BC,
∴ `P_BV_B^γ = P_CV_C^γ`
`P_C = P_B(V_B/V_C)^γ`
= `P_B(1/2)^γ`
= `2^(-γ)P_B`
Similarly, `P_D = 2^(-γ)P_A`
Total work done by the engine in one cycle ABCDA
`W = W_(AB) + W_(BC) + W_(CD) + W_(DA) = W_(BC) + W_(DA)`
= `((P_CV_C - P_BV_B))/(1 - γ) + ((P_AV_A - P_DV_D))/(1 - γ)`
`W = 1/(1 - γ) [2^(-γ) P_B (2V_B) - P_BV_B + P_AV_A - 2^(-γ) P_B (2V_B)]`
= `1/(1 - γ) [P_AV_B (2^(-γ + 1) - 1) - P_AV_A (2^(-γ + 1) - 1)`
= `1/(1 - γ) (2^(1 - γ) - 1) (P_B - P_A)V_A`
= `3/2 [1 - (1/2)^(2/3)](P_B - P_A)V_A`
d. Heat (Q) is supplied to the engine only from A to B. Thus Q = ΔQAB = ΔUAB = nCVΔT
= `3/2 R(T_B - T_A)` .....[As CV = (3/2)R and ΔT = TB – TA]
= `P_CV_C^γ = P_BV_B^γ`,
`P_C = P_B(V_B/V_C)^γ`
= `P_B(1/2)^γ`
= `P_B2^-γ` .....(As VB/VC = 1/2)
Thus, `P_CV_C^γ = (P_B2^-γ)(2V_B) = 2^(1 - γ)P_BV_B`
= `3/2 (P_BV_B - P_AV_A)`
= `3/2 (P_B - P_A)V_A` .....(PV = RT and VB = VA)
The efficiency of the engine,
η = `W/Q = (3/2 [1 - (1/2)^(2/3)] (P_B - P_A)V_A)/(3/2 (P_B - P_A)V_A)`
= `[1 - (1/2)^(2/3)]`
APPEARS IN
संबंधित प्रश्न
Find the change in the internal energy of 2 kg of water as it is heated from 0°C to 4°C. The specific heat capacity of water is 4200 J kg−1 K−1 and its densities at 0°C and 4°C are 999.9 kg m−3 and 1000 kg m−3 respectively. Atmospheric pressure = 105 Pa.
Answer the following in one or two sentences.
State the first law of thermodynamics.
For a particular reaction, the system absorbs 8 kJ of heat and does 2.5 kJ of work on its surrounding. What will be the change in internal energy of the system?
An electric appliance supplies 6000 J/min heat to the system. If the system delivers a power of 90 W. How long it would take to increase the internal energy by 2.5 × 103 J?
The V cc volume of gas having `γ = 5/2` is suddenly compressed to `(V/4)` cc. The initial pressure of the gas is p. The final pressure of the gas will be ______.
Derive an expression for the work done during an isothermal process.
In an adiabatic process, ______.
What is Isobaric process?
Explain the formulation of first law of thrmodynamics.
Calculate work done when 2 moles of ideal gas expands by 5 dm3 isothermally at pressure 1.2 bar.
