Advertisements
Advertisements
प्रश्न
A gun can fire shells with maximum speed v0 and the maximum horizontal range that can be achieved is R = `v_0^2/g`. If a target farther away by distance ∆x (beyond R) has to be hit with the same gun (Figure), show that it could be achieved by raising the gun to a height at least `h = Δx[ 1 + (Δx)/R]`
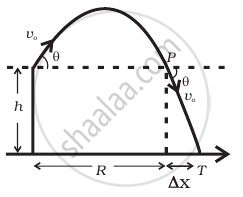
उत्तर
This problem can be approached in two different ways:
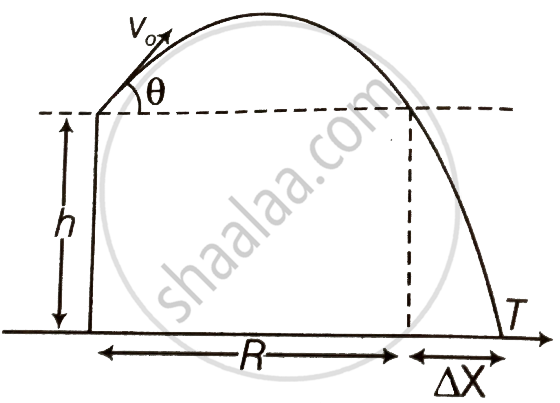
- Referring to the diagram, target T is at a horizontal distance x = R + Δx and between the point of projection y = – h.
- From point P in the diagram projection at speed v0 at an angle θ below horizontal with height h and horizontal range ΔxA)
Applying method (i)
Maximum horizontal range, `R = v_0^2/g`, for θ = 45° ......(i)
Let the gun be raised through a height h from the ground so that it can hit the target. Let vertically downward direction is taken as positive
The horizontal component of initial velocity = v0 cos θ
The vertical component of initial velocity = – v0 sin θ
Taking motion in vertical direction, `h = (- v_0 sin theta) t + 1/2 "gt"^2` ......(ii)
Taking motion in the horizontal direction, `(R + Δx) = v_0 cos θ xx t`
⇒ `t = (R + Δx)/(v_0 cos θ)` ......(iii)
Substituting the value of t in equation (ii), we get
`h = (- v_0 sin θ) xx ((R + Δx)/(v_0 cos θ)) + 1/2 g ((R + Δx)/(v_0 cos θ))^2`
`h = - (R + Δx) tan θ + 1/2 g (R + Δx)^2/(v_0^2 cos^2 θ)`
As angle of projection is θ = 45°, therefore
`h = - (R + Δx) + tan 45° + 1/2 g (R + Δx)^2/(v_0^2 cos^2 45^circ)`
`h = - (R + Δx) xx 1 + 1/2 g (R + Δx)^2/(v_0^2 (1/2))` ......`(∵ tan 45^circ = 1 and cos 45^circ = 1/sqrt(2))`
`h = - (R + Δx) + (R + Δx)^2/R` ......[Using equation (i), R = `"v"_0^2`/g]
= `- (R + Δx) + 1/R (R^2 + Δx^2 + 2RΔx)`
= `- R - Δx + (R + (Δx^2)/R + 2Δx)`
= `Δx + (Δx^2)/R`
`h = Δx(1 + (Δx)/R)`
Hence proved.
APPEARS IN
संबंधित प्रश्न
The ceiling of a long hall is 25 m high. What is the maximum horizontal distance that a ball thrown with a speed of 40 m s–1 can go without hitting the ceiling of the hall?
Show that the projection angle `theta_o` for a projectile launched from the origin is given by
`theta_o =tan^(-1) ((4h_m)/R)`
Where the symbols have their usual meaning
A car starts from rest and accelerates at 5 m/s2. At t = 4 s, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at t = 6 s? (Take g = 10 m/s2)
Two particles are projected in air with speed vo at angles θ1 and θ2 (both acute) to the horizontal, respectively. If the height reached by the first particle is greater than that of the second, then tick the right choices
- Angle of projection: q1 > q2
- Time of flight: T1 > T2
- Horizontal range: R1 > R2
- Total energy: U1 > U2
A boy throws a ball in air at 60° to the horizontal along a road with a speed of 10 m/s (36 km/h). Another boy sitting in a passing by car observes the ball. Sketch the motion of the ball as observed by the boy in the car, if car has a speed of (18 km/h). Give explanation to support your diagram.
A fighter plane is flying horizontally at an altitude of 1.5 km with speed 720 km/h. At what angle of sight (w.r.t. horizontal) when the target is seen, should the pilot drop the bomb in order to attack the target?
A body is thrown from a point with speed 50 m/s at an angle 37° with horizontal. When it has moved a horizontal distance of 80 m then its distance from point of projection is ______.
A circular disc of radius r = 5m is rotating in horizontal plane about y-axis. Y-axis is vertical axis passing through the centre of disc and x-z is the horizontal plane at ground. The height of disc above ground is h = 5 m. Small particles are ejecting from disc in horizontal direction with speed 12 m/s from the circumference of disc then the distance of these particles from origin when they hits the x-z plane is:
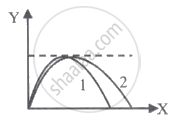
The trajectories of the two projectiles are shown in the figure. Let T1 and T2 be the time periods and u1 and u2 be their speeds of projection. Then ______.
A ball is projected from the ground with a speed 15 ms-1 at an angle θ with horizontal so that its range and maximum height are equal, then tan θ will be equal to ______.
