Advertisements
Advertisements
प्रश्न
A land is in the shape of rhombus. The perimeter of the land is 160 m and one of the diagonal is 48 m. Find the area of the land.
उत्तर

Perimeter of the rhombus = 160 m
4 × side = 160
Side of a rhombus = `160/4`
= 40 m
In ΔABC, a = 40 m, b = 40 m, c = 48 m
s = `("a" + "b" + "c")/2`
= `(40 + 40 + 48)/2 "cm"`
= `128/2`
= 64 m
s – a = 64 – 40 = 24 m
s – b = 64 – 40 = 24 m
s – c = 64 – 48 = 16m
Area of the ΔABC = `sqrt(64 xx 24 xx 24 xx 16)`
= 8 × 24 × 4
= 768 sq.m
Since ABCD is a rhombus Area of two triangles are equal.
Area of the rhombus ABCD = (768 + 768) sq.m
= 1536 sq.m
∴ Area of the land = 1536 sq.m
APPEARS IN
संबंधित प्रश्न
Find the area of a quadrilateral ABCD in which AD = 24 cm, ∠BAD = 90° and BCD forms an equilateral triangle whose each side is equal to 26 cm. (Take √3 = 1.73)
Find the area of an equilateral triangle having each side x cm.
If each side of a triangle is doubled, the find percentage increase in its area.
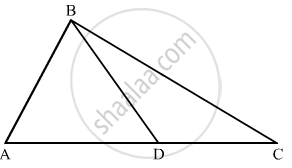
In the given figure, the ratio AD to DC is 3 to 2. If the area of Δ ABC is 40 cm2, what is the area of Δ BDC?
A square and an equilateral triangle have equal perimeters. If the diagonal of the square is \[12\sqrt{2}\] cm, then area of the triangle is
A park is in the shape of a quadrilateral. The sides of the park are 15 m, 20 m, 26 m and 17 m and the angle between the first two sides is a right angle. Find the area of the park
The length of each side of an equilateral triangle having an area of `9sqrt(3)`cm2 is ______.
The edges of a triangular board are 6 cm, 8 cm and 10 cm. The cost of painting it at the rate of 9 paise per cm2 is ______.
In a triangle, the sides are given as 11 cm, 12 cm and 13 cm. The length of the altitude is 10.25 cm corresponding to the side having length 12 cm.
