Advertisements
Advertisements
प्रश्न
A lawn is in the shape of a semicircle of diameter 42m. the lawn is surrounded by a flower bed of width 7m all round. Find the area of the flower bed in m2.
उत्तर

There are two concentric semi circles. The diameter of the inner circle = 42 or radius, a = 21m. The radius of the outer circle, b = 21 + 7 = 28m.
Because the radius of a Circle with diameter d is r = `"d"/(2)`
The Area of the inner Semi-circle with radius a = `(pi"a"^2)/(2) = (pi21^2)/(2)`
∴ The Area of the outer Semi-circle with radius b = `(pi"b"^2)/(2) = (pi28^2)/(2)`
and The Area of a Semi-circle with radius r = `(pi"r"^2)/(2)`
The Area of the flower bed
= `(pi28^2)/(2) - (pi21^2)/(2)`
= `pi/(2)(28^2 - 21^2)`
= `pi/(2)(784 - 441)`
= `pi/(2)(343)`
= `(22)/(7 xx 2)(343)`
= 539m2.
APPEARS IN
संबंधित प्रश्न
Find the cost of polishing a circular table-top of diameter 1.6 m, if the rate of polishing is ₹ 15/m2. (Take π = 3.14)
Find the area and perimeter of an isosceles right angled triangle, each of whose equal sides measure 10cm.
AB is a chord of a circle with centre O and radius 4 cm. AB is of length 4 cm. Find the area of the sector of the circle formed by chord AB.
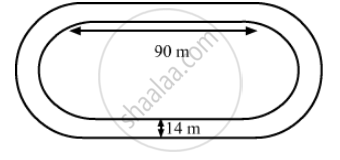
The inside perimeter of a running track (shown in the following figure) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If the track is everywhere 14 m wide. find the area of the track. Also find the length of the outer running track.
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. (Use π = 22/7).
In the following figure, the boundary of the shaded region consists of four semi-circular arcs, the smallest two being equal. If the diameter of the largest is 14 cm and of the smallest is 3.5 cm, find
the length of the boundary.
the area of the shaded region.

From a thin metallic piece, in the shape of a trapezium ABCD, in which AB || CD and ∠BCD = 90°, a quarter circle BEFC is removed (in the following figure). Given AB = BC = 3.5 cm and DE = 2 cm, calculate the area of the remaining piece of the metal sheet.
If diameter of a circle is increased by 40%, then its area increase by
From a rectangular sheet of paper ABCD with AB = 40 cm and AD = 28 cm, a semicircular portion with BC as diameter is cut off. Find the area of the remaining paper.
On increasing the diameter of a circle by 40%, its area will be increased by
