Advertisements
Advertisements
प्रश्न
A man sitting at a height of 20 m on a tall tree on a small island in the middle of a river observes two poles directly opposite to each other on the two banks of the river and in line with the foot of the tree. If the angles of depression of the feet of the poles from a point at which the man is sitting on the tree on either side of the river are 60° and 30°respectively. Find the width of the river.
उत्तर
Let BC be the width of the river. And the angles of depression on either side of the river are 30° and 60° respectively. It is given that AC = 20 m.
Let BC = x and CD = y. And ∠ABC = 30°, ∠ADC = 60°.
Here we have to find the width of the river.
We have the corresponding figure as follows

So we use trigonometric ratios.
In a triangle ABC
`=> tan B = (AC)/(BC)`
`=> tan 30^@ = 20/x`
`=> 1/sqrt3 = 20/x`
`=> x = 20sqrt3`
Again in a triangle ADC
`=> tan D = (AC)/(CD)`
`=> tan 60^@ = 20/y`
`=> sqrt3 = 20/y`
`=> y = 20/sqrt3`
`=> x + y = 20sqrt3 + 20/(20sqrt3)`
`=> x + y = 80/sqrt3`
Hence width of river is `80/sqrt3` m
APPEARS IN
संबंधित प्रश्न
When the sun is 30º above the horizontal, Find the length of shadow cast by a building 50m high
The elevation of a tower at a station A due north of it is α and at a station B due west of A is β. Prove that the height of the tower is `\frac{AB\sin \alpha \sin \beta }{\sqrt{\sin^{2}\alpha -\sin ^{2}\beta `
A statue, 1.6 m tall, stands on a top of pedestal, from a point on the ground, the angle of elevation of the top of statue is 60° and from the same point the angle of elevation of the top of the pedestal is 45°. Find the height of the pedestal.
The length of a string between a kite and a point on the ground is 90 meters. If the string makes an angle O with the ground level such that tan O = 15/8, how high is the kite? Assume that there is no slack in the string.
The angle of elevation of an aeroplane from a point on the ground is 45° after flying for 15seconds, the elevation changes to 30° . If the aeroplane is flying at a height of 2500 meters, find the speed of the areoplane.
The angle of depression of a car, standing on the ground, from the top of a 75 m high tower, is 30°. The distance of the car from the base of the tower (in m.) is:
A kite is flying at a height of 30 m from the ground. The length of string from the kite to the ground is 60 m. Assuming that there is no slack in the string, the angle of elevation of the kite at the ground is
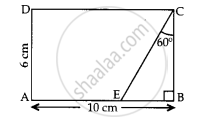
In given figure, the value of CE is ____________.
Two poles are 25 m and 15 m high, and the line joining their tops makes an angle of 45° with the horizontal. The distance between these poles is ______.
From the top of a 7 m high building, the angle of elevation of the top of a cable tower is 60° and the angle of depression of its foot is 30°. Determine the height of the tower.
