Advertisements
Advertisements
प्रश्न
A park is in the form of rectangle 120m × 100m. At the centre of park there is a circular lawn. The area of park excluding lawn is 8700m2. Find the radius of circular lawn.
उत्तर

Dimensions of rectangular park length = 120m
Breadth = 100m
Area of park = l × b
= 120 × 100 = 12000𝑚2.
𝐿𝑒𝑡 𝑟𝑎𝑑𝑖𝑢𝑠 𝑜𝑓 𝑐𝑖𝑟𝑐𝑢𝑙𝑎𝑟 𝑙𝑎𝑤𝑛 𝑏𝑒 𝑟
Area of circular lawn = 𝜋𝑟2
Area of remaining park excluding lawn = (area of park) – (area of circular lawn)
⇒ 8700 = 12000 – 𝜋r2
⇒ 𝜋r2 = 12000 – 8700 = 3300
⇒`22/7× r^2 = 3300`
⇒ `r^2` = 150 × 7 = 1050
⇒ `r = sqrt(1050) = 5sqrt(42)` 𝑚𝑒𝑡𝑟𝑒𝑠
∴ radius of circular lawn = 5`sqrt(42)` metres.
APPEARS IN
संबंधित प्रश्न
From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in the given figure. Find the area of the remaining portion of the square [Use Π = 22/7]

In the given figure, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touches externally two of the remaining three circles. Find the area of the shaded region. [Use Π = 22/7]

Calculate the area of the designed region in the given figure common between the two quadrants of circles of radius 8 cm each. [Use Π = 22/7]

The perimeter of a right triangle is 40 cm and its hypotenuse measure 17 cm. Find the area of the triangle.
The length of the two sides of a right triangle containing the right angle differ by 2 cm. If the area of the triangle is 24xm^2, find the perimeter of the triangle.
If the area of an equilateral triangle is `81sqrt3 cm^2` find its height.
Find the area of shaded region in Fig. 4, where a circle of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm. (Use π = 3.14 and\[\sqrt{3}\] =1.73)
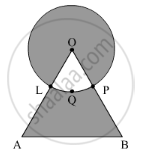
In Figure 5, ABCD is a quadrant of a circle of radius 28 cm and a semi circle BEC is drawn with BC as diameter. Find the area of the shaded region ?\[[Use\pi = \frac{22}{7}]\]
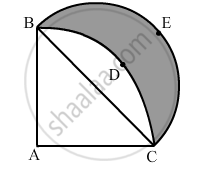
In Fig. 7, PQ and AB are respectively the arcs of two concentric circles of radii 7 cm and
3.5 cm and centre O. If ∠POQ = 30°, then find the area of the shaded region. [User`22/7`]
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km away on time, it has to increase its speed by 250 km/hr from its usual speed. Find the usual speed of the plane.
